By Nami Youn




 
 

Write-up  #9

Pedal triangels
 

Introduction

In this write-up, I will investigate a Pedal point and a pedal triangle. First, I will make a script for the general construction of a pedal triangle RST using GSP. Then, I try to explore some situations; If the pedal point is the centroid of a triangle ABC, then what happens? If the podal point is the incenter or circumcenter of a triangle ABC.


1. Constrution

Given a triangle ABC, if we pick a point(P) in the plane, we can construct the perpendicular line to each side through the given point. The Pedal triangle(RST) can be constructed by intersections of these perpendicular lines and each side. The point P is called a pedal point.

If you would like to see the script  for construct this pedal triangle, click here.


2. Examining the details

1) If P is the Incenter of the triangle....





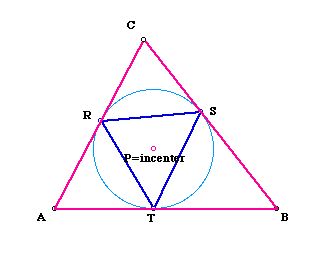
The pedal triangleSTR is always inside the given triangle ABC. It is reason that the Incenter ithe point of concurrency of the angle bisectors and is inside of triangle ABC. The pedal triangle is located in the incircle of triangle ABC.
This incircle is also the circumcircle of triangle RST.
 

If you would like to see the GSP file, click here.


2) If P is a Centroid of the triangle.....

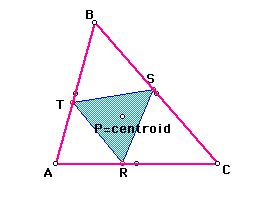
It is clear that the pedal triangle STR is always inside the triangle ABC.
PT, PR and PS are the bisectors of each side because P is the centroid of ABC and PT, PR and PS are perpendicular to AB, BC, and AC, respectively, because P is a pedal point. Therefore, P is the circumcenter of ABC.

If you would like to see the GSP file, clickhere.


3)  If P is a Circumcenter of the triangle.....




When the pedal point is located at the circumcenter of triangle ABC, the points R, S, T occur at the midpoints of triangle
ABC's sides. Also, pedal triangle is always the medial triangle of triangle ABC. It is reason that the circumcenter lies on the perpendicular bisector of each side. Theresforem when we drops the perpendicular to each side of the triangle from this circumcenter, it intersects the triangle at the midpoints.
 

If you would like to see the GSP file, click here.

To the top

Return to Nami's Home Page