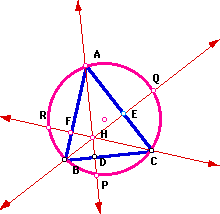
Construct any acute triangle ABC and its Circumcircle. Construct the three altitudes AD, BE, and CF. Extend each altitude to its intersection with the Circumcircle at corresponding points P, Q, and R.

What can you say about the following ratio?
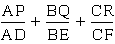
One conjecture might be that HD is congruent to DP. Let's try and prove that now by trying to show congruent triangles.

Similarly, we can show that HF is congruent to FR, and HE is congruent to EQ.
Now, back to the ratio..... 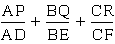 .
.
Let first take apart the equation and look at just one element,
say (AP/AD.) First of all, AP= AD + DP.
So by substituting, we get (AP/AD) = (AD + DP/AD) = 1 + (DP/AD).
Similarly, we have
(BQ/BE) = (BE + EQ/BE) = 1 + (EQ/BE), and (CR/CF) = (CF + FR/CF)
= 1 + (FR/CF)
Adding them up we get:
(1 + (DP/AD)) + (1 + (EQ/BE)) + (1 + (FR/CF)) = 3 + ((DP/AD) +
(EQ/BE) + (FR/CF)).
Yet we have already proven that DP ? HD, EQ ? HE, and FR ? HF.
So the latter part of the above equation becomes
(HD/AD) + (HE/BE) + (HF/CF) . What does this equal? Recall the
area of a triangle being A = (1/2)base *height.
So the A(Triangle ABC) = (1/2)(AD * BC). Solving for AD: AD =
(2*A(Triangle ABC)/BC).
And the A(Triangle CBH) = (1/2)(HD * BC). Solving for HD: HD =
(2*A(Triangle CHB)/BC).
Therefore, (HD/AD) = (A(Triangle CHB)/A(Triangle ABC)). We can
figure out the values of the other two ratios in a similar
manner. Our equation then becomes:
(HD/AD) + (HE/BE) + (HF/CF) = (A(Triangle CHB)/A(Triangle ABC))
+ (A(Triangle CHA)/A(Triangle ABC)) + (A(Triangle AHB)/A(Triangle
ABC)) = 1 because the sum of area of the
smaller triangles is equal to the area of the larger triangle.
Hence our final equation then becomes: