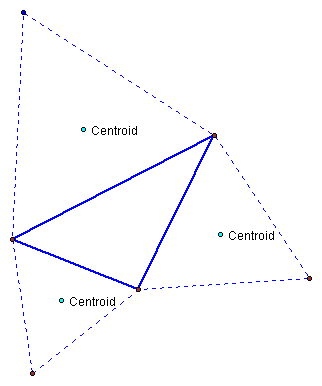
Given any triangle, construct equilateral triangles on all three sides of the triangle as shown below.

If we connect the centroids to form a triangle (shown in yellow), this triangle known as Napoleon's Triangle is always equilateral. Although this triangle is named after Napoleon Bonaparte, it is not known whether he actually discovered the triangle or not. Some say that one of his friends actually discovered it and named it after him. However, we do know that Napoleon Bonaparte was interested in mathematics.

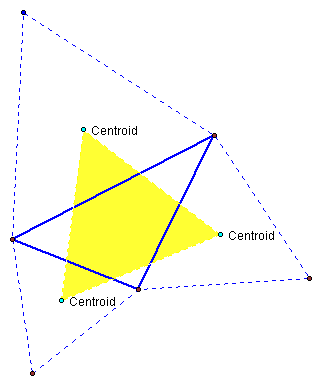
A Construction Proof:

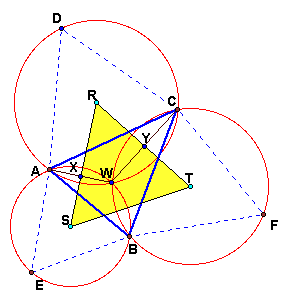
Prove that Triangle RST is equilateral.

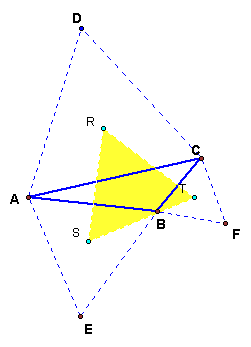
Construct circumcircles around the equilateral triangles with segments AW and WC drawn as shown.

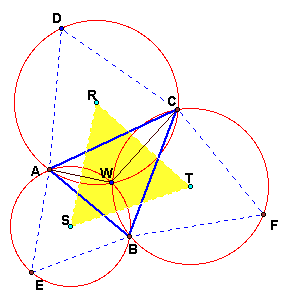
Angle AWC = 120 degrees since opposite angles of quadrilaterals inscribed in a circle are supplementary. We also know that RS is perpendicular to AW because a line segment joining the centers of two intersecting circles is perpendicular to the common chord.

We now have a quadrilateral RXWY of which we know 3 of the angle measures. Angle RXW = 90, Angle RYW = 90, Angle AWY = 120. Therefore, Angle R = 60 degrees. If we do this same construction for each triangle, we will find that angels T and S also equal 60 degrees. Therefore Triangle RST is equilateral.

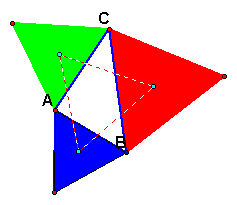
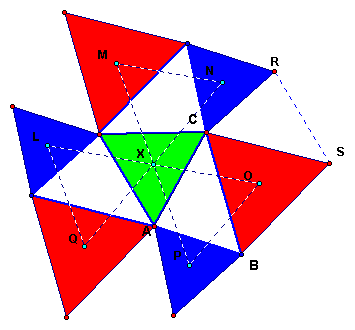
If we rotate Napolean's Triangle around the center of one of the equilateral triangles, we have the following diagram.

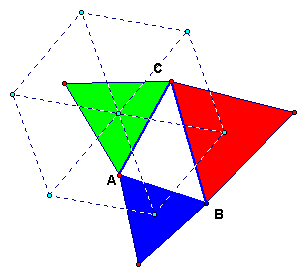
This appears to be a regular hexagon which means that the angles are 60 degrees.

If we rotate the entire figure 120 degrees and then 240 degrees around one of the equilateral triangles (I chose the green one), we will have the following:

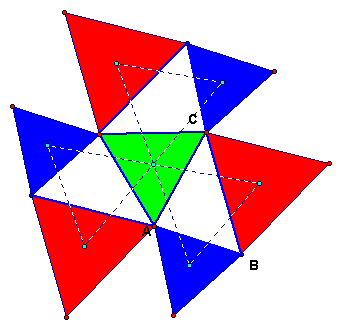
We know that each triangle is the above figure is equilateral simply because of the rotational symmetry.

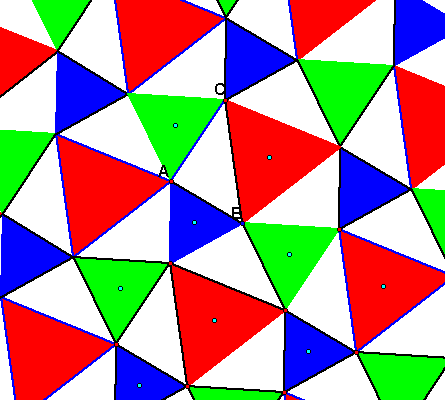
Because the above proof, we know we can tessellate the plane with this geometric figure. I have done this using iterative tool in GSP to continue rotating each figure until the plane is filled as show below.

Click here to see the Tesselation using GSP. (Use your '+' and '-' keys to show the tessellation.)

RETURN to Phyllis' home page