
Recall, the equation of a circle:

One set of parametric equations for the circle are given by
x=a cos t
y=b sin t
When a=1 and b=1, the graph is a circle with radius=1. Since:

Here's a graph if you don't believe me!


If we change a, the circle becomes an ellipse intersecting the x-axis at a and -a and intersecting the y-axis at -1 and 1.
Let, a = 4 and b =1
So, we'll have an ellipse intersecting the x-axis at -4 and 4 and intersecting the y axis at -1 and 1.


If we change b the circle becomes an ellipse intersecting the x-axis at 1 and -1 and intersecting the y-axis at -b and b.
Let, a = 1 and b = 3
So, we'll have an ellipse intersecting the x-axis at -1 and 1 and intersecting the y-axis at -3 and 3.


The normal form of an ellipse is the following implicit equation:

The axes of this ellipse are the x and y axis, a and b are the axis lengths, and the larger one of a and b is the major axis while the smaller one is the minor axis.
Hyperbolas? What's that you utter in anticipation?
The normal form of a hyperbola is the following implicit equation:

The definition of major axis and minor axis are identical to that of ellipses. The x-axis intersects the curve at two points (a,0) and (-a,0) and the y-axis does not intersect the curve at all. A possible parametric form of the above hyperbola is the following
x=a sec t
y= b tan t
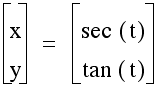
Has the following graph:

The x-axis intersects the curve at two points (1,0) and (-1,0) and the y-axis does not intersect the curve at all.


The x-axis intersects the curve at two points (3,0) and (-3,0) and the y-axis does not intersect the curve at all.
Alas, I digress. Back to circles!
Now, if add 3 to a unit circle with radius 2, what happens?


The unit circle moves 3 units to the right on the x-axis and 3 units upward in the y-axis.
What if?


Yes, you guessed it! The circle moves 1 unit to the right on the x-axis and 3 unit upward on the y-axis.
I'll leave it to the reader to explore at will. No, no, please hold your applause. Remember, I love you.