Assignment # 10
Fall 2002 Semester
Sketchpad and Graphing Calc - Parametric Equations - Curves
Number 10-7: Write the parametric equations of a line through (7,5) with a slope of 3.
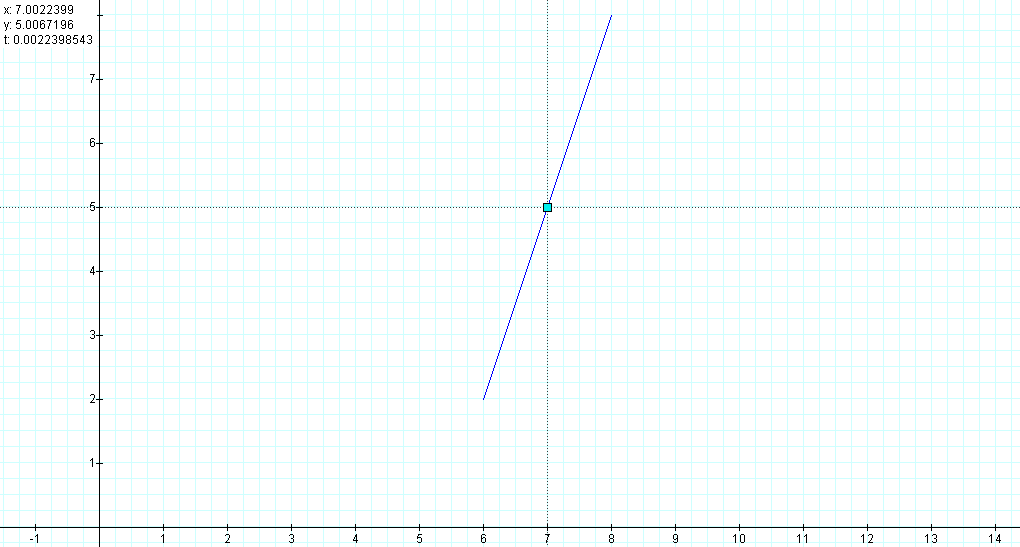
The following graph shows the line with the point at the y-intercept.
To determine the parametric equations, let's start with the basic equation for a line:
y = mx + b.
Adding the slope of 3, and known points:
When x = 7, then y = 5
We get: 5 = 3 * 7 + b; which yields b (y-intercept) = -16.
Now to transfer this into a parametric equation, start with:
x = t + 7 for t = -1 to +1
In this case, the center point (t = 0) will be at the location (7,5).
Substituting "t+7" for x in the original equation and solving for y yields:
y = 3(t+7) - 16
y = 3t + 5
The parametric equations are listed below.
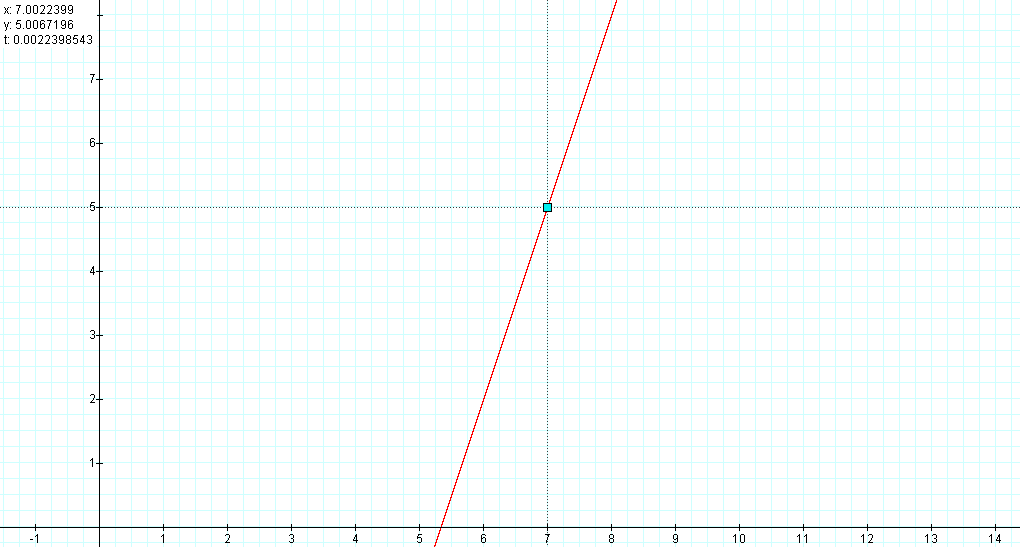
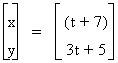The graph for t = -1
through +1 is shown below:
