Assignment # 2

The purpose of this assignment is to explore the quadratic equation. We begin with the following equation:

y = ax² + bx + c.

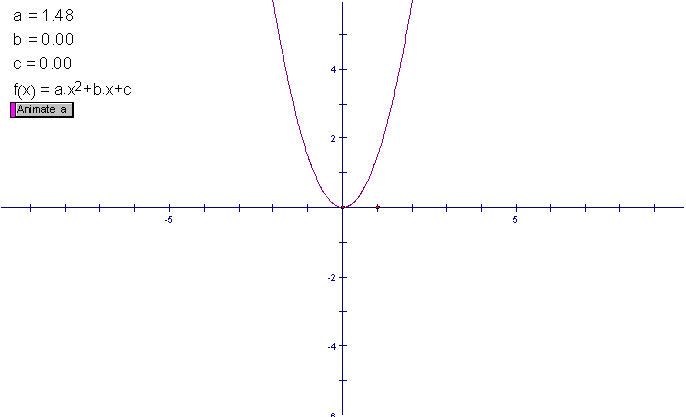
We examine this equation by holding two of the parameters constant and varying the third. We first hold b=0 and c=0 and vary parameter a. The following GSP sketch shows that all these parabolas have the same locus but as a increases the parabola “stretches” or becomes narrower. This occurs because the distance between the locus and the directrix becomes smaller and smaller. The same occurs when a is negative except the parabola “stretches” as the absolute value of a gets larger. Therefore a changes the shape but not the location of the parabola. Click here to see this animation in GSP.

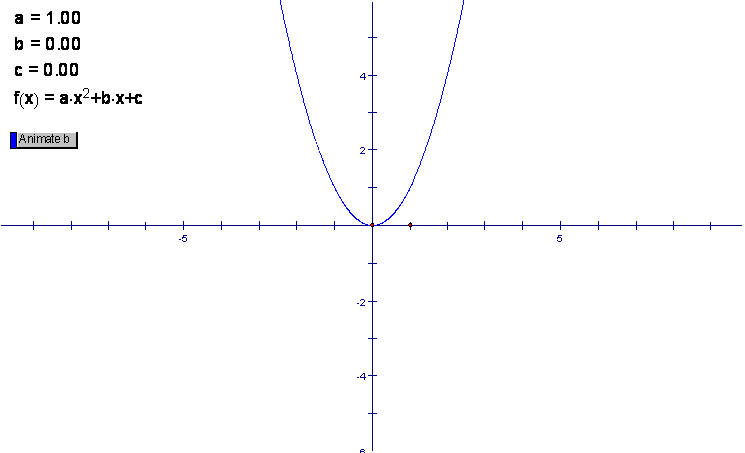
Now we can examine the effect of b on the quadratic equation by varying b and holding a=1 and c=0. We can see in the following GSP sketch that b changes the roots of the equation (that is when it crosses the x-axis). As b gets larger one of the roots of the parabola becomes smaller. Similarly when b is negative and the absolute value of b gets larger, one of the roots gets larger. Yet we notice that all the parabolas have one root in common. Therefore b moves the parabola up and down while simultaneously moving it from side to side yet never changes the distance from the locus to the directrix. Therefore b does not change the parabola itself only its location. Click here to see this animation in GSP.

Now we can examine the effect of c on the quadratic equation. By holding a=1 and b=0 by varying c we obtain the following GSP sketch. Click here to see this animation in GSP.

We can see that c changes the y-intercept of the equation. As c gets larger the y-intercept gets larger. We also notice that all the parabolas have the same shape and therefore the distance from the locus to the directrix remains constant. Therefore c only moves the parabola up and down and does not change the shape. Similarly to b, we can say that c only changes the location.