

The centroid (G) of a triangle is the common intersection of three medians. A median of a triangle is the segment from the vertex to the midpoint of the opposite side. The centroid of the triangle will change as the triangle changes but there will always be a centroid.

To see an animation of this picture click here (GSP)
The orthocenter (H) of a triangle is the common intersection of the three lines containing the altitudes. An altitude is a perpindicular segment from a vertex to the line of the opposite side.

For an aninmation of this picture click here (GSP)
The circumcenter (C) of a triangle is the point in the plane equidistant from the three vertices of the triangle. Since a point equidistant from two points lies on the perpendicular bisector of the segment determined by the two points, C is on the perpendicular bisector of the segment determined by the two points, C is on the perpendicular bisector of each side of the triangle.

For an illustration of this construction click here (GSP)
The Incenter (I) of a triangle is the point on the interior of the triangle that is equidistant from the three sides. Since a point interior to an angle that is equidistant from the two sides of hte angle lies on the angle bisector, then I must be on the angle bisector of the triangle.
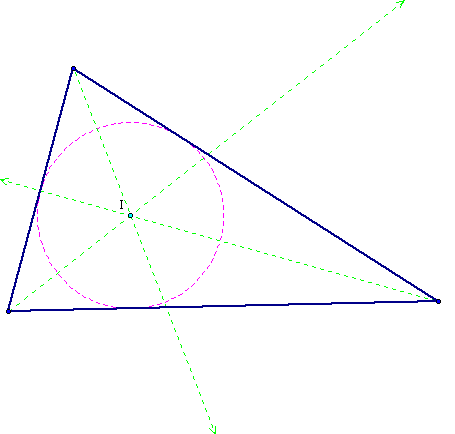
For an illustration of this click here (GSP)
Now taking the four triangles above and combining them into one triangle the figure appears like
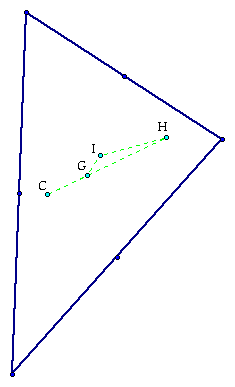
When the Four Triangles are combine the incenter, orthocenter, and centroid form an inner triangle. The orthocenter and centroid also form a line with the circumcenter. The also does exist one point where the four points are all the same point forming a center in the triangle. There is also a point where the four form one triangle and another where they form a line. Click here to examine more (GSP).
When a triangle is taken and another triangle is drawn in the middle connected with the midpoint, and then the four centers are connected with the small and large triangle the points and triangle formed appear as so:
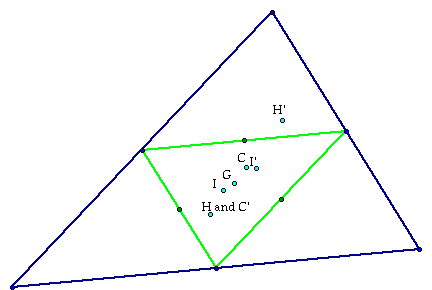
The ( ' ) is represented points on the larger triangle. G has no prime because the point for G and G' is the same. To examine this triangle more closely click here (GSP).
When an acute triangle is taken inside the triangle and the four points are drawn the triangle would appear as:
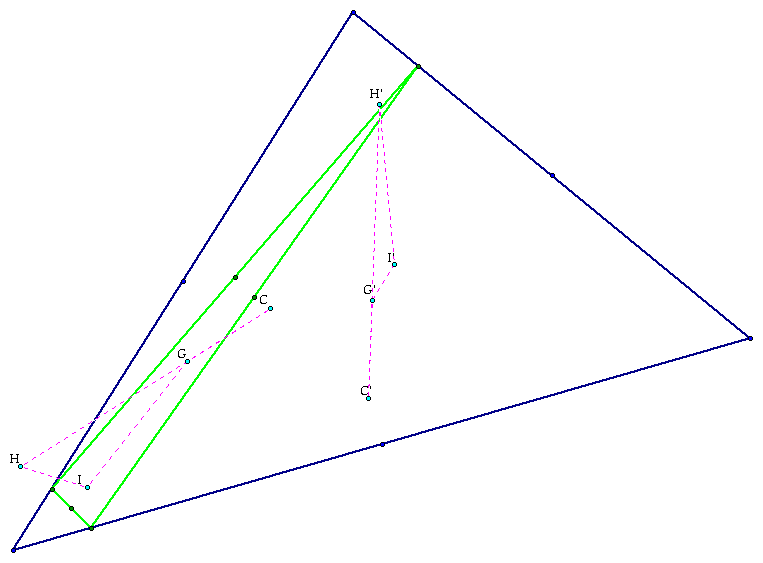
Click Here to see this triangle in GSP
When a right triangle is taken insides the triangle the four points are drawn on the orginal triangle and appear as so:
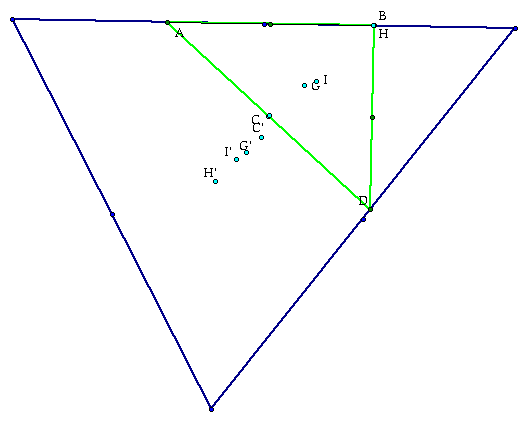
To examine this further click here (GSP)
When an obtuse triangle is drawn inside the triangle, the four points are drawn on the orginal triangle and appear as so:
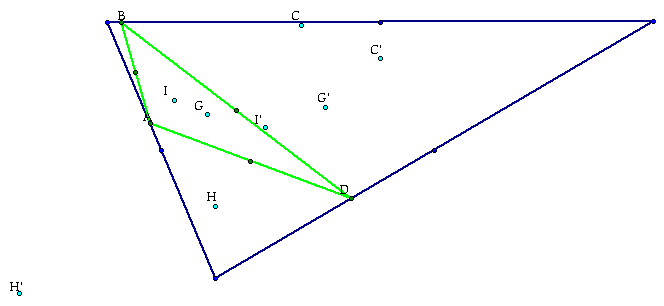
To examine this further click here (GSP)
For the explanation of how I plan on using click here