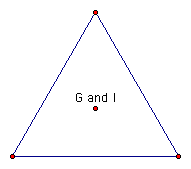
To start, we will look at some of the properties of triangles by themselves to get familiar with them. First, the centroid (G) of a triangle is the point at which the three medians of the triangle intersect.
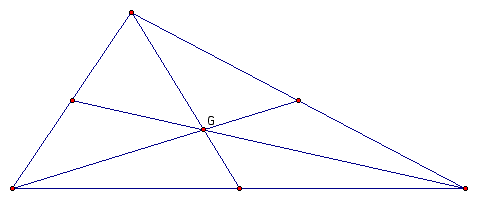
Next, the orthocenter (H) is the point where the three altitudes of the triangle intersect. An altitude is the line from a vertex which is perpendicular to the opposite side. The orthocenter does not necessarily have to be in the interior of the triangle.

The circumcenter (C) of a triangle is the concurrence point of the perpendicular bisectors of the triangle. These are lines perpendicular to the sides which intersect each side at its median. This circumcenter does not have to be in the interior of the triangle either.
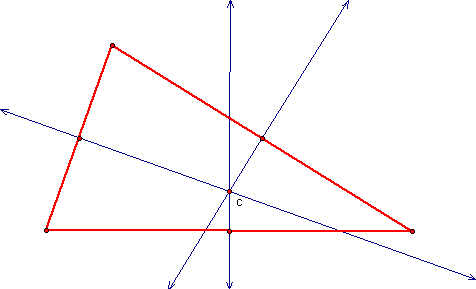
If we wanted to find a circle that contained all three vertices of a triangle, this circumcenter is the center of that circle, and we could draw a radius to each vertex.

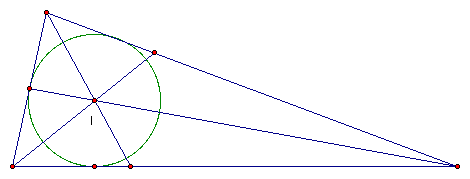
Finally, the incenter (I) of a triangle is the point in the interior where all three angle bisectors intersect. The incenter creates the center of what is called the incircle, which is a circle that touches all three sides at exactly one point. To find the radius, we must draw a perpendicular line from I to any side since that will be the shortest distance to any of the sides.
All four of these are different centers of traingles. Let's look at all four of them together on the same triangle.
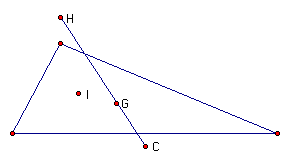
It is interesting to see that the centroid, orthocenter and circumcenter appear to be collinear here. It turns out that these three points will always be collinear for any three points we pick to be the vertices of a triangle. The incenter can be on that line as well, but it obviously does not have to be on it.
The traingle above seems to have an obtuse angle, so let's look at an acute triangle to see these properties again.
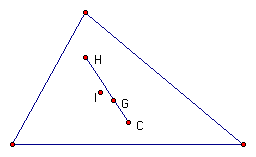
With an acute triangle, all four of these centers will be in the interior, but when we have an obtuse angle as one of the angles, the circumcenter and orthocenter will move to the exterior. They will remain collinear, though.
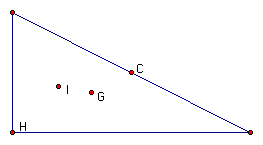
A right triangle is an interesting case. The orthocenter will be at one of the vertices, and the circumcenter will be the at the midpoint of the opposite side.
One other interesting case to look at is an equilateral triangle. The centroid and the incenter will be at the same point.