Objectives:
1. to know multiple definitions of slope
2. to be able to find the slope of a line given rise and run or two points
3. to be able to apply the concept of slope to real world situations
Definitions:
Slope:
1) the change in y divided by the change in x.
2) rise divided by run
Note: These definitions are exactly the same. They just use different terminology to say the same thing.
Slope represents how a line changes as it goes upwards or downwards. It can be positive, negative, zero, or undefined. The last two are special cases that occur when we have either horizontal or vertical lines. The first two are the most common and will be discussed first.
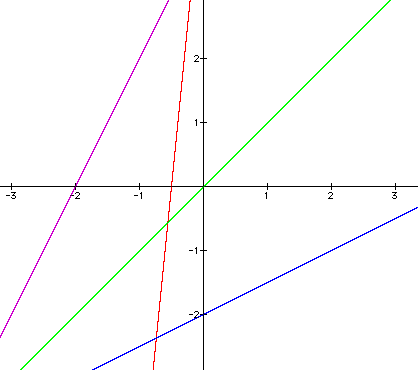
A line with a positive slope goes upwards from left to right. A line with a positive slope can have any real number value greater than zero. Some examples of lines that would have positive slopes can be seen in the next picture.

Notice that some lines are steeper than the others, but that they all go upwards from left to right. So, the slopes of the lines are all postive yet different.
A line with a negative slope goes downward from left to right. A line with a negative slope can have any real number value less than zero. Some examples of lines that have a negative slope can be seen in the next picture.
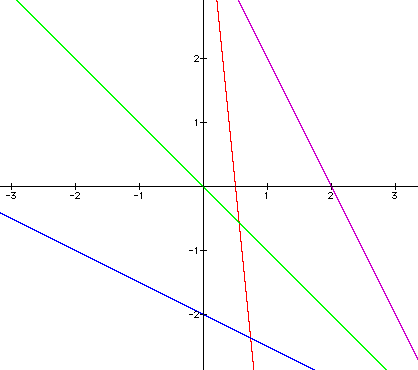
Again, notice that some lines are steeper than the others, but that in this case they all go downwards. Here, the slopes of the lines are all negative, yet different.
The first of the special cases is the horizontal line. The slope of any horizontal line is zero. As you will see later, it is zero because for any two given points on the line, they have the same y-value. Horizontal lines are of the form y = any number, and when they are graphed, they are flat or go just left and right. Some examples of horizontal lines can be seen below.

The second special case is the vertical line. The slope of any vertical line is undefined. It is undefined because for any two given points on the line the points have the same x-value. Vertical lines are of the form x = any number, and when they are graphed, they go straight up and down. Some examples of vertical lines can be seen below.
Now that we understand the four different types of slopes, it is time to be able to find the value of slope. In order to find slope which will be represented by m, we use the following equation.
slope (m) = y2 - y1 / x2 - x1
Where x1 , y1, x2, and y2 are the coordinates of two points written as (x1 , y1) and (x2, y2).
or sometimes it is thought of as: rise/run where rise is an up or down movement and run is a left or right movement. Remember, that on a computer "/" means divided by which is also a fraction bar. So, slope is usually written as a fraction is lowest terms and not as decimal. It is kept as a fraction to make it easier to graph.
We will look at how to find slope using rise and run first because it is the easier of the two. It is also the less common of the two to use because it is rare to actually have the rise and the run of a line. So, when given rise and run, we just make our fraction and reduce as can be seen in examples 1 and 2.
Example1: Find the slope of the following lines using the given information.
a) rise = 4, run = 3. So, the slope is the fraction 4/3.
b) run = 7, rise = 5. So, the slope is the fraction 5/7.
c) rise = -12, run = 10. So, the slope is fraction -12/10 which reduces to -6/5.
Remember, leave slopes as a fraction. DO NOT change them into a decimal.
Example2: Find the slope of the following lines using the given information.
a) rise = 0, run = 5. So, the slope is the fraction 0/5 which is 0. Therefore, this must be a horizontal line.
b) rise = 2, run = 0. So, the slope is the fraction 2/0 which is undefined becasue it is impossible to divide by 0. Therefore, this must be a vertical line.
Pay close attention to the difference between these two special cases. Students tend to get them confused.
It is now time to find the slope of a line given two points of the form (x1 , y1) and (x2, y2). So, we will now be using the equation: slope (m) = y2 - y1 / x2 - x1 . Here again, we will leave our answers in the form of a fraction in lowest terms. It is a three step process to find slope.
1. Substitute for x1 , y1, x2, and y2.
2. Subtract.
3. Reduce the fraction. (if possible).
Examples 3-5 will illustrate how to find the slope of a line given 2 points.
Example3: Find the slope of a line given 2 points.
a) (4, 6) and (5, 8). Substituting gives us (8 - 6)/(5 - 4). Now subtract to get the fraction, 2/1 which reduces to 2. So, the slope is 2.
b) (3, 7) and (11, 5). Substituting gives us (5 - 7)/(11 - 3). Now subtract to get the fraction -2/8 which reduces to -1/4.
Example 4: Find the slope of a line given 2 points.
a) (8, -2) and (-6, -5). Substituting gives us (-5 - - 2)/(-6 - 8). Subtract to get the fraction -3/-14 which reduces to 3/14.
b) (-3, - 5) and (-7, -1). Substituting gives us (-1 - -5)/(-7 - -3). Subtract to get the fraction 4/-4 which reduces to -1.
Example 5: Find the slope of a line given 2 points.
a) (2, 6) and (-4, 6). Substituting gives us (6 - 6)/(-4 - 2). Subtract to get the fraction 0/-6 which reduces to 0. Therefore, this must be a horizontal line.
b) (-8, 4) and (-8, 2). Substituting gives us (2 - 4)/(-8 - -8). Subtract to get the fraction -2/0 which is undefined because we can't divide be zero. So, this must be a vertical line.
Have you noticed any patterns? Is there a simple way to tell when a slope is zero or undefined? You should now be able to find the slope of line given the rise and run or given two points. Make sure to keep track of signs. This is where the most mistakes occur.