

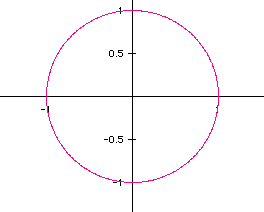
Here, we start with a and b equal 1 which gives the equations: x = cos (t) and y = sin (t). The graph of these equations is a cirlce with a radius of 1 which can be seen below.
Now, Let's increase a and b while keeping them equal and see what happens. This increase will keep making graphs of concentric circles with radii equal to a and b. This can be seen graphically in the picture below which has values of a and b up to 4.

Now, it is time to see what happens when a < b. This will change the graph from a circle to an ellipse with the y-axis as the major axis and the x-axis as the minor axis. Also, the major axis will be the length of b and and the minor axis will be the length of a. Some examples of this can be seen in the next graph.

The final scenario in this section is what happens when a > b. This is the opposite of the a< b scenario. Here, we again get graphs of ellipses, but now the x-axis is the major axis and the y-axis is the minor axis. Some examples of this can be seen in the following graph.

The generalized patterns involved in this section are as follows. If a=b, then the graph is a circle with radius equal to a. If a < b or a > b, then the graph is an ellipse with the major axis that coincides with the larger a or b value.