


Here, just like in section one of this write-up, we will start with the basic scenario when a = b = 1. When this is graphed, it creates a line segment that starts at y = 1 and ends at x = 1. As a and be are increased but remain equal, the graphs continue to be segments that go from y = b to x = a. All of the graphs are in the first quadrant because the exponent is two which makes all values of x and y positive. A graph with the values of a = b from one to four is below.

The next scenario to look at is when a < b. Here again, we get segments and remain in quadrant one due to the fact that the exponent is still two. The differnce is that the endpoints do not have the same value. Now, the endpoint of the segment on the y-axis will be equal to b, and the endpoint of the segment on the x-axis will be equal to a with b always being greater than a. Some examples of this with b values up to four and a values less than the chosen b value can be seen below.
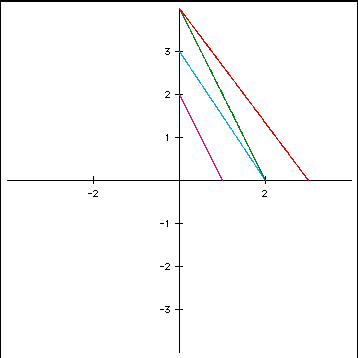
The last part of section two deals with a > b. This works just like a < b, except it reversed. Again, we get segments in the firt quadrant with endpoints on the x and y axes. The only difference is that the endpoint on the x-axis will be larger than the endpoint on the y-axis. Some examples of this can be seen in the following picture.
