

For this part of the final, we will use the following diagram
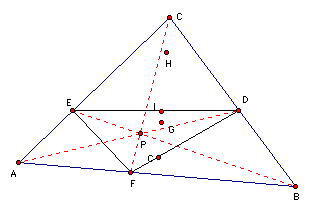
to show that the ratio of triangle ABC to triangle DEF is greater than or equal to 4. Again, we use GSP to make all of our calculations and find the initial ratio which is 4.26. So, we get a value that was expected.
Now, we can move point p anywhere on the inside of the triangle and see that the ratio is always greater than or equal to 4. Then, we change the size of the triangle by moving the vertices and the ratio is still greater than or equal to 4. Click here to open a GSP sketch that will allow you see this for yourself.
The truly interesting aspect of this is finding when the ratio is exactly 4. So, I proceeded to use a script from assignment 5 that creates the triangle centers. They can be seen on the diagram above labeled H, I, G, and C. Once we have the centers, it is easy to drag point p on top of the centers to see if one of them gives the desired ratio.
I started having P = H and found the following
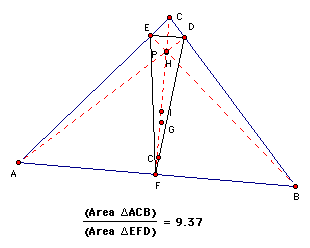
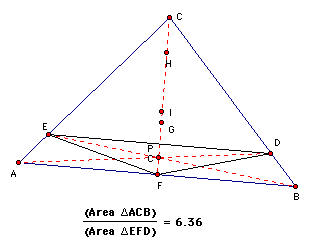
which is not 4. So, then I tried P = I
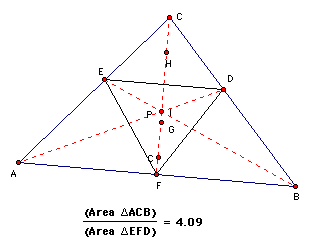
which was very close but not equal to 4. Next, I checked P = C
which was worse. So then, that leads one to believe that the ratio of 4 is between G and C, so finally I let P = G and found
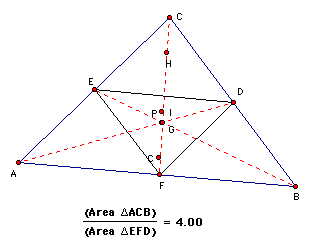
which is exactly what we are looking for. Therefore, the ratio of the two triangles equals 4 when the point on the interior of triangle ABC is the centroid.