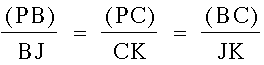Consider any triangle ABC. Select a point P inside the triangle and draw lines AP. BP, and CP extended to their intersections with the opposite sides in points D, E, and F, respectively.

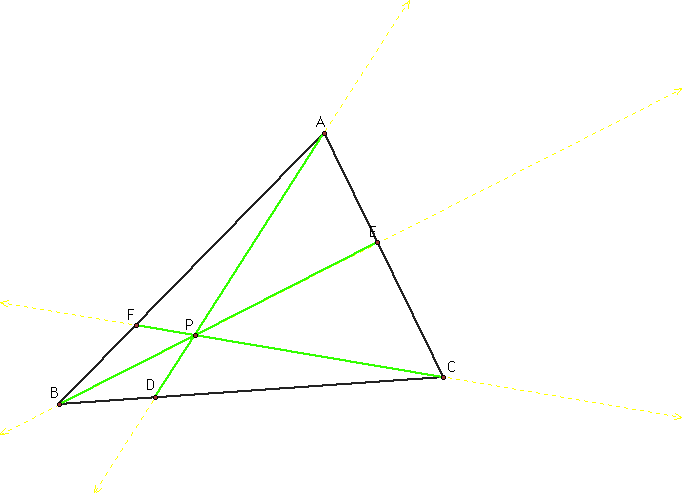
Explore (AF), (BD), (EC), and (FB), (DC), (EA) for various triangles and various locations of P.

What kinds of assumptions can we make by observing this image?

Let's look at this construction on an obtuse triangle.

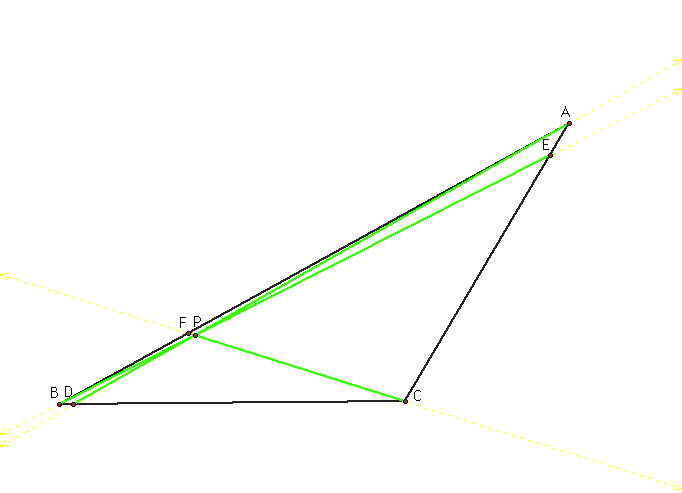
Have you noticed anything to discuss?

Let's look at this construction one more time on a right triangle.

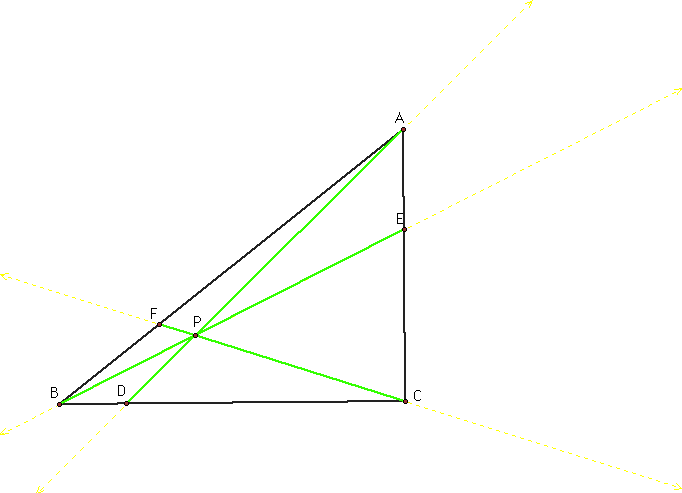
Let's see if we can find any similar triangles.

We constructed the line segments FC, AD, and BE through the same point P, so we know that all three lines intersect at the same point. What conclusion can we make from this statement?

Since the two lines intersect at the same point, angles FPD and APC are vertical angles; therefore they are congruent. Angles FPA and CPD are also vertical angles, so they, too, are congruent. Angles BPD and EPA are congruent. Let's look at our triangle with the congruent angles indicated.

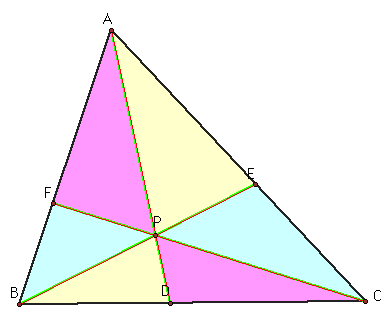
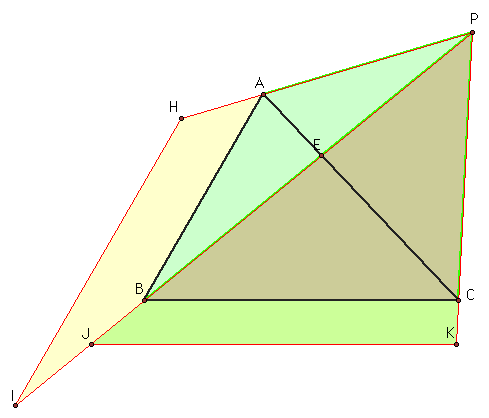
Let's examine some ratios:

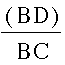
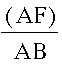
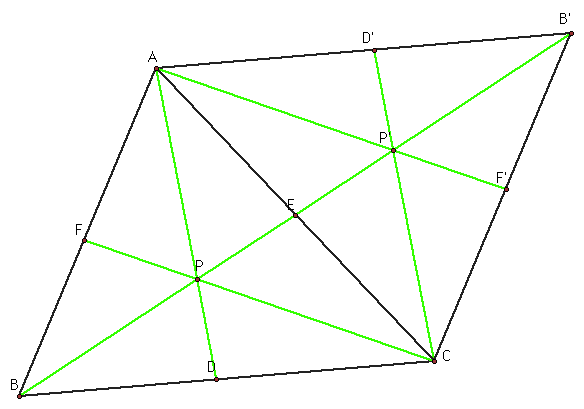
Segment BE was extended to BJ, and segment AD was extended to AK. Next, two lines were constructed parallel to BE, (HI and LC), and two lines were constructed parallel to AD, (HL and IC). Since segments AK and BJ intersect, angles APB and KPJ are vertical triangles, so they are congruent.

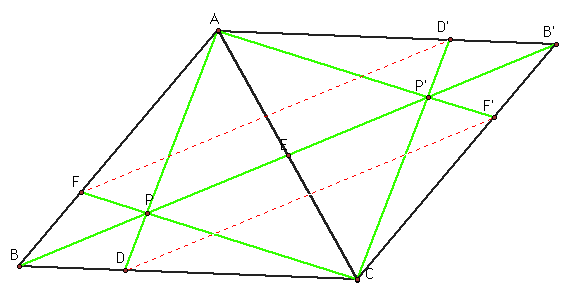
The original triangle was rotated 180 degrees to create a parallelogram.

As we can see by connecting the points FD' and DF', we have created similar triangles.Triangle FAT is similar to triangle BAE, and triangle PAE is similar to triangle DAG. It looks like we need more similar triangles. Let's construct a parallel line to BC and a parallel line to AD.

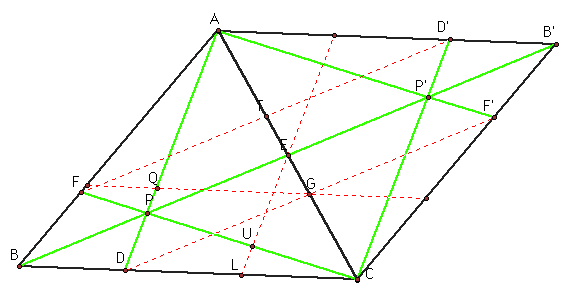
Now, we can see more similar triangles. Triangle LCU is similar to triangle DCP, triangle UCE is similar to triangle PCA, and triangle QAC is similar to triangle DAC.

What do these similar triangles show us?

Since triangle FAT is similar to triangle BAE,

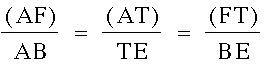
Since triangle PAE is similar to triangle DAG,

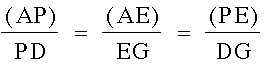
Since triangle DBP is similar to triangle LBE,

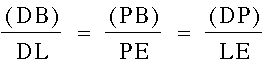
Since triangle LCU is similar to triangle DCP,

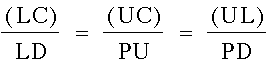
Since triangle UCE is similal to triangle PCA,

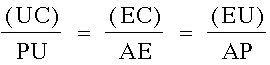
Since triangle QAC is similar to triangle DAC,

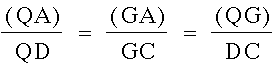
By Cevian's Theorem AF*BD*CE = 1

............................FB*DC*EA

Triangle BFP is similar to triangle CEP, triangle PAE is similar to triangle DPB, and triangle DPC is similar to triangle FPA.

What if point p is outside the triangle?

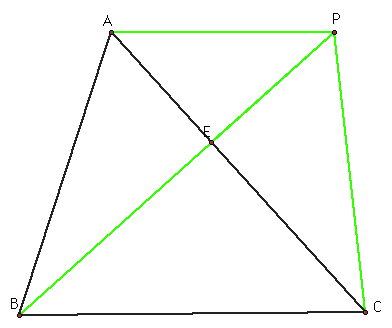
Are any of the triangles similar?

Segment PA was extended to PH, segment PB was extended to PJ and PI, and segment PC as extended to segment PK. Segment HI is parallel to segment AB, and segment JK is parallel to segment BC. Now triangle APB is similar to triangle HPI, and triangle BPC is similar to triangle JPK.

Since triangle BPC is similar to triangle JPK,

 

Since triangle APB is similar to triangle HPI,

Can we make any conclusions?

Does it matter where P is outside of the triangle?

To see how the triangles change as P moves around the circumcircle click here.

 

It appears as though the triangles outside of traingle ABC are similar as P moves around the circumcircle.

 

 

RETURN