Assignment 8: Altitudes and Orthocenters
by
Mike Cotton
This assignment examines the relationship between
altitudes and orthocenters.
Let's begin by constructing a triangle ABC.
Next, let's construct the orthocenter of triangle ABC.
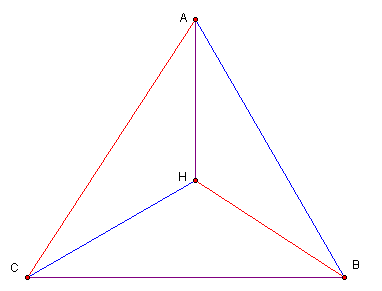
Figure 2
Now, let's construct the orthocenters of triangles HBC, HAB, & HAC.
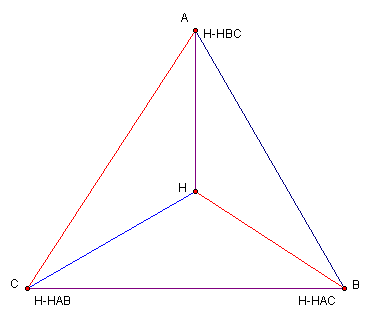
Figure 3
Note that point A is the orthocenter of triangle HBC, point B is the
orthocenter of triangle HAC, and point c is the orthocenter of triangle
HAB. But why?
Let's explore triangle HBC with its orthocenter H-HBC
at point A.
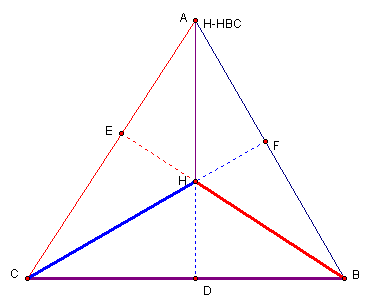
Figure 4
The ORTHOCENTER (H) of a triangle is the common intersection of the
three lines containing the altitudes. An altitude is a perpendicular
segment from a vertex to the line of the opposite side. (Note: The foot
of the perpendicular may be on the extension of the side of the
triangle.)
To explain why the orthocenter of triangle HBC coincides with point A
lets review the construction of orthocenter H. The alitude of vertex A
is segment AD. The altitude of vertex B is segment BE, and the alitude
of vertex C is segment CF.
Now looking at triangle HBC.
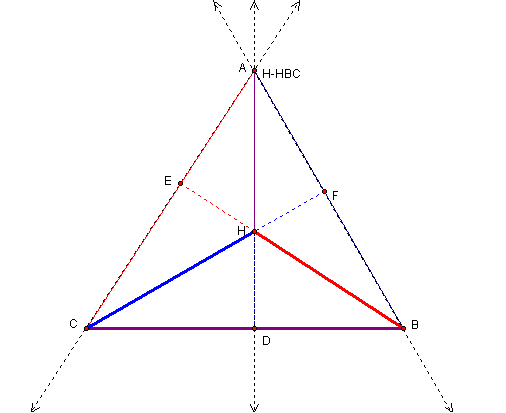
Figure 5
By construction, the altitude for vertex H (line AH) is the same
altitude for vertex A, so the orthocenter for triangle will lie on line
AH. Also by previous construction, line AB is perpendicular to segment
CH, so line AB is the altitude for vertex B of triangle HBC. And again
by previous construction, line AC is perpendicular to segment BH, so
line AC is the altitude for vertex C of triangle HBC. Lines AH, AB
& AC all intersect at point A, so it follows that the orthocenter
of triangle HBC (H-HBC) is the point A.
This same argument would prove that B (H-HAC) is the orthocenter of
triangle HAC, and C (H-HAB) is the orthocneter of triangle HAB.