for
c=-4, -2, 0, 2, and 4.I expect for this function to be a parabola, and shifted up or down on the y-axis. Let's see!
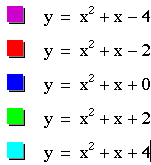
I was right! As you can see the graph is in the shape of a parabola, but shifted it is shifted up when the value of c is positive and down when the value of c is negative.
for a=-4, -3, -2,
-1, 0, 1, 2, 3, and 4.I expect for this to again be a parabola, but for the width to vary, and for the parabola to flip upside down when a is negative. Let's see!
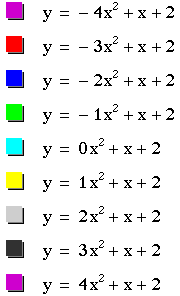
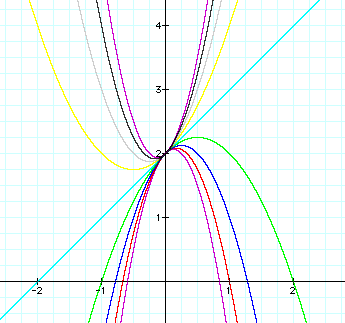
This is not exactly as I expected. The parabolas are changing in width, but they are also shifting along the line y=x+2. Also, the line y=x+2 is one of functions for when a=2.
In general, when observing a function, if the constant that you are varying is in front of an x, the graph will vary in width, and if the constant that you are varying is added to the function, the graph will shift up or down. Unless, of course the function is linear. Then, if the constant is multiplied in front of the x, then the slope changes, not the width.