A parabola is the set of points equidistant from a line, called the directrix, and a fixed point, called the focus. Assume the focus is not on the line. Construct a parabola given a fixed point for the focus and a line (segment) for the directrix.

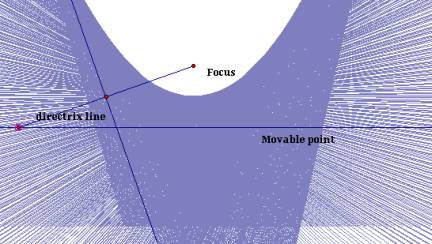
1. In order to begin construction, we must first make a line and a point of Focus. We should call the line, the “directrix line”. The point should be labeled as the focus.

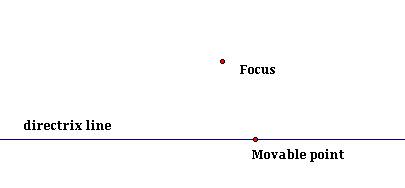
2. Next, make a movable point on the directrix line. This point will help us to trace the path of the parabola.

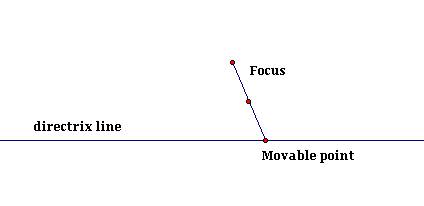
3. Now, we can construct a segment from the focus to the movable point. We must also find a point that is equidistant from the focus to the movable point. We can construct this point by finding the midpoint of the segment. Clicking the CONSTRUCT button at the top of the screen and choosing the MIDPOINT option can create the midpoint.

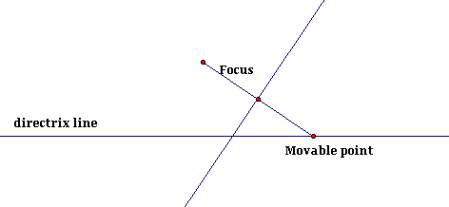
4. The midpoint can now be used to construct a perpendicular bisector through the segment from the movable point to the focus. The perpendicular bisector is representative of one of the tangent lines in our parabola.

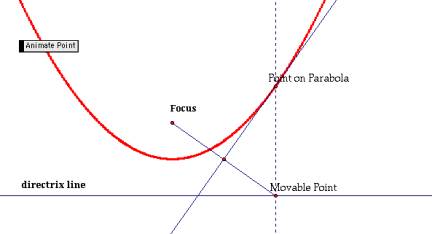
5. By tracing the perpendicular bisector and animating the movable point we are able to see our parabola being formed.

________________________________________________________

Our construction above proves that all points on a parabola are equidistant from the directrix and the focus. Let’s look closer at finding a point on the parabola. By making a line of intersection with our movable point, tangent line and directrix line, we can find a point on our parabola.

In order to operate the animation yourself, press here.

Other Explorations:

Let’s investigate the construction of ellipses:

An ellipse is the set of all points whose sum of its distance from two foci is a constant.

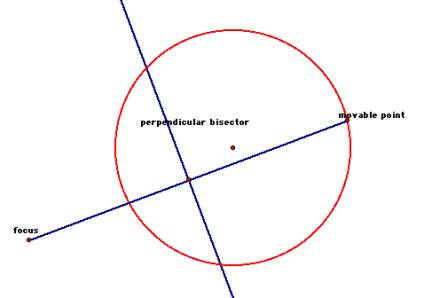
1. We must construct a circle (directrix) and a point (focus).

2. Now, make a movable point on the directrix circle by highlighting the circle and construct a point on the circle.

Draw a segment from the focus to the movable point on the circle and create the perpendicular bisector of this segment. The perpendicular bisector acts as one of the tangent lines in our ellipse.

3. Next, in order to see all tangent lines of our ellipse, we can

trace the perpendicular bisector and animate the movable point.

View the animation, by clicking here.

Notice that the center and the focus are the foci of our ellipse.

_____________________________________________________

Let’s, construct a hyperbola:

A hyperbola is the locus of all points, P, in the plane the difference of whose distances from two fixed points separated by a distance 2c is a given constant.

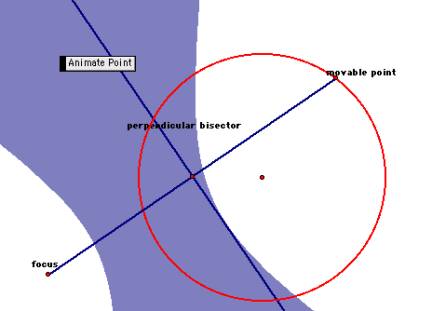
1. Similarly to our ellipse construction, we can construct our

hyperbola by creating a focus, circle and a movable point on the circle. Next, we can construct a segment that joins the movable point and the focus. Finding the midpoint of the constructed segment and creating a perpendicular line through the midpoint and the constructed segment can make the perpendicular bisector.

2. By animating the movable point and tracing the

perpendicular bisector, we can see our hyperbola being developed.

Click to see a complete animation of the hyperbola.

This concludes our look at parabolas and other conic explorations.

___________________________________________________

Return to Homepage