Fibonnaci Sequence
By
Audrey V.
Simmons
The Fibonnaci Sequence is a most amazing sequence of numbers
that appears in different problems and is related to the golden ratio of 
or
1.618034. We will look at how
these two prevalent items come together.
PART A: Calculate the Fibonnaci Sequence/Determine Ratios
The Fibonnaci Sequence is a sequence of numbers that is calculated by adding the previous two numbers to get the next value. For example,
F(0) = 1, f(1)=1, f(2) = 2, f(3) = 3, f(4) = 5,…f(n) = f(n-1) + f(n-2).
These numbers are represented in column A. To find the numbers in column C (B was skipped to make the numbers more readable), take the ratio of adjacent terms. A3/A2 = 2, A4/A3 = 1.5, A5/A4 = 1.66666667, and so on.
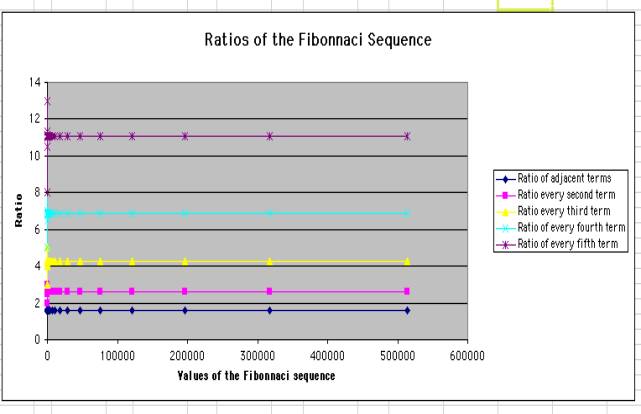
The ratio of the numbers becomes the golden ratio.
Let’s try the ratio of every second term to determine if there is any relationship.
The ratio of every second term is the golden number plus one.
Would the next ratio be the golden ratio plus two?
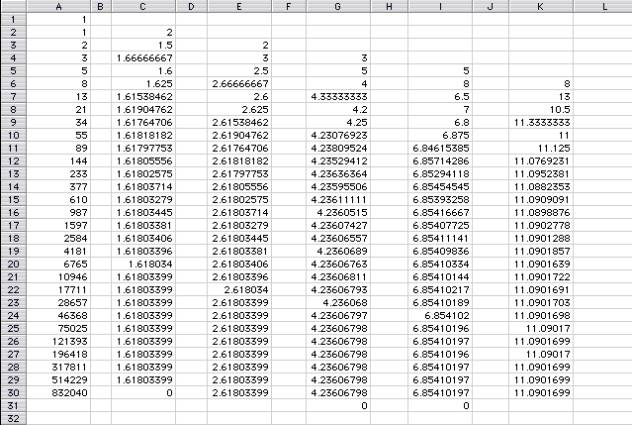
If we look at column G which represents the ratio of every third term, we can see that 4.23606798 is not the golden ratio plus two. However, it is the golden ratio times two plus one.
In column I when we find the ratio of every fourth term the answer approaches the number 6.85410197. The golden ratio times three plus two.
Column K(ratio of every fifth term), the number 11.0901699 is the golden ratio times 5 plus three.
Below is a graph of the respective ratios showing the constant nature of the amounts.
PART B: Arbitrary
numbers and their ratios
This time random numbers have been picked to begin the sequence, but we have used the same formula f(n+1) = f(n) +f(n-1) to calculate our numbers.
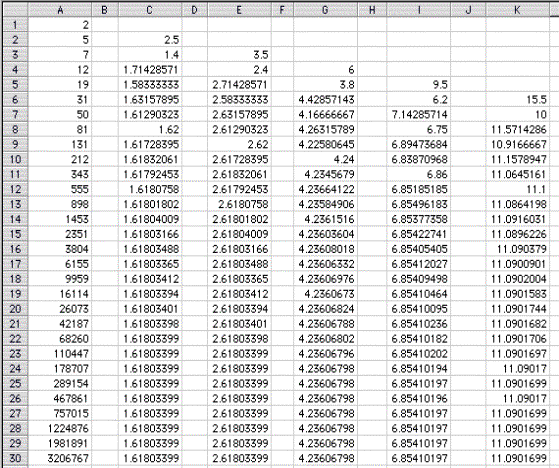
Even if the initial numbers are different, when the Fibonnaci formula is used to calculate the terms the respective ratios reach the same values as the values in the Fibonnaci ratios.