6. Take any triangle. Construct a triangle connecting the three midpoints of the sides. This is called the MEDIAL triangle. It is similar to the original triangle and one-fourth of its area. Construct G, H, ,C and the I for this new triangle. Compare to G,H, C, and I in the original triangle.

First, I constructed a triangle using GSP. I labeled the three points A,B and C. Notice that segment AB is green, segment BC is blue and CA is red.

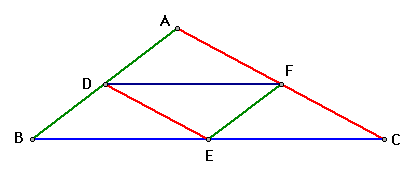
Next, I constructed the midpoints of the three sides of the triangle. Notice that D is the midpoint of segment AB, E is the midpoint of segment BC and F is the midpoint of segment CA. Now I can connect the three points to make a triangle referred to as the medial triangle. The medial triangle is one-fourth of the area of the original triangle ABC. Also, the lengths of the sides in the medial triangle are one-half the length of their corresponding side in the original triangle ABC.

After constructing the medial triangle, I wanted to explore and find out if the properties of a medial triangle can be seen visually. In other words, I wanted to see if the area of the medial triangle is really 1/4 of the area of triangle ABC and that the lengths of the sides in the medial triangle are 1/2 the length of the corresponding sides in triangle ABC.

First, I had to find the heights of the two triangles. For triangle ABC, I took the point A and line segment BC to construct the perpendicular line. For triangle EFD, I took point E and line segment FD to construct the perpedicular. Then I found the heights by measuring the lengths of AG and HE. I also found the lengths of all the sides of the medial triangle and the original triangle ABC.

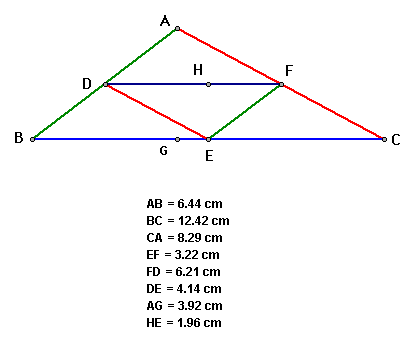
Now, using the formula for the area of a triangle, I can compare the areas of the two triangles. Remember, Area=(1/2)(Base)(Height)

The area of triangle ABC is 1/2(BC)(AG). 1/2(12.42)(3.92)=24.3432

The area of triangle EFD is 1/2(FD)(HE).

1/2(6.21)(1.96)=6.0858

Is 6.0858=1/4(24.3432)?

Yes it is, so the area of the medial triangle is one-fourth of the area of triangle ABC.

Finally, I compared the side lengths of the original triangle ABC and medial triangle EFD. Notice that the corresponding sides appear to be parallel and that I labeled them with the same colors so that it would be easier to make comparisons.

Compare DE and CA.

Is 4.14=1/2(8.29)?

4.14=4.145...Yes

Compare EF with AB.

Is 3.22=1/2(6.44)?

3.22=3.22....Yes

Compare FD with BC.

Is 6.21=1/2(12.42)?

6.21=6.21....Yes

Since all the sides of the medial triangle are 1/2 of the length of the sides in the original triangle ABC, it appears that both properties of the medial triangle have now been met. Therefore, we can say that triangle EFD is, indeed, a medial triangle of triangle ABC.

Return