

Objective: Apply the definitions of the median and the altitude of a triangle and the perpendicular bisector of a segment. State and apply related theorems.
Definitions:
Perpendicular Bisector Theorem:
If a point lies on the perpendicular bisector of a segment, then the point is equidistant from the endpoints of the segment.

Converse of the Perpendicular Bisector Theorem:
If a point is equidistant from the endpoints of a segment, then the point lies on the perpendicular bisector of the segment.

Angle Bisector Equidistant Theorem:
If a point lies on the bisector of an angle, then the point is equidistant from the sides of the angle.

Converse of the Angle Bisector Equidistant Theorem:
If a point is equidistant from the sides of an angle, then the point lies on the bisector of the angle.
Exercises:
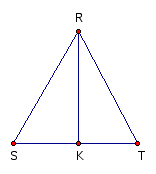
Triangle RST is needed for Exercises1-5
1. If K is the midpoint of ST, then RK is called a(n)_______ of triangle RST.
2. If RK is perpendicular to ST, then RK is called a(n) ________ of triangle RST.
3. If K is the midpoint of ST and RK perpendicular ST, then RK is called a(n)
________ of ST.
4. If RK is both an altitude and a median of Triangle RST, then:
a. Triangle RSK congruent Triangle RTK by __________.
b. Triangle RST is a(n) _________ triangle.
5. If R is on the perpendicular bisector of ST, then R is equidistant from _______ and _________. Thus ______=_______.
6. Prove the Perpendicular Bisector Theorem.
Given: The line through point X is the perpendicular bisector of BC; A is also on this line.
Prove: AB=AC
7. Prove the Converse of the Perpendicular Bisector Theorem.
Given: AB=AC
Prove: A is on the perpendicular bisector of BC.
Plan for proof: The perpendicular bisector of BC must contain the midpoint of BC and be perpendicular to BC. Draw an auxiliary line containing A that has one of these properties and prove that it has the other property as well. For example, first draw a segment from A to the midpoint X of BC. You can show that AX is perpendicular to BC if you can show that Angle 1 congruent to Angle 2. Since these angles are corresponding parts of two triangles, first show that triangle AXB is congruent to triangle AXC.
8. Prove the Angle Bisector Equidistant Theorem.
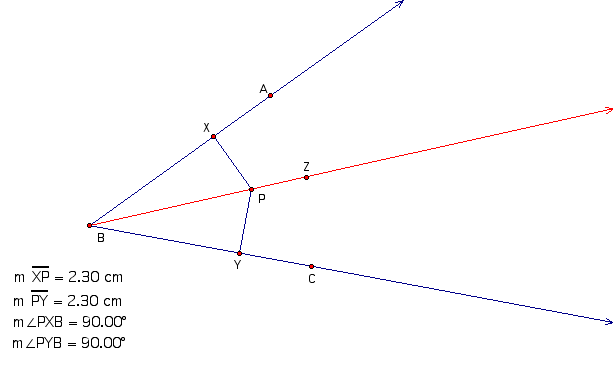
Given: BZ bisects Angle ABC; P lies on BZ; PX is perpendicular to BA; PY is perpendicular to BC
Prove: PX=PY
9. Prove the converse of the Angle Bisector Equidistant Theorem.
Given: PX perpendicular to BA; PY perpendicular to BC; PX=PY
Prove: Ray BP bisects Angle ABC