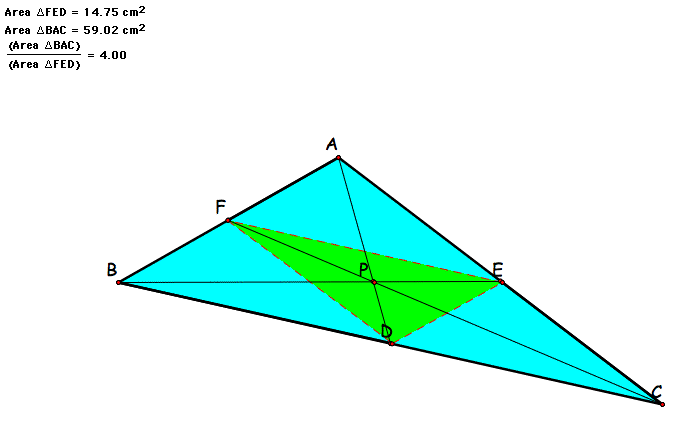
A. Consider any triangle ABC. Select a point P inside the triangle and draw lines AP, BP, and CP extended to their intersections with the opposite sides in points D, E, and F respectively.
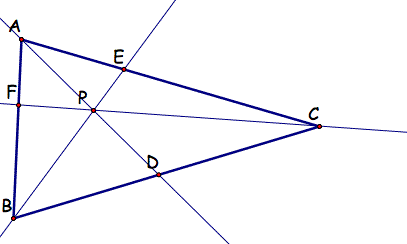
Explore (AF)(BD)(EC) and (FB)(DC)(EA) for various triangles and various locations of P.

What do you see in the picture above? The products are equal!
To use a dynamic GSP document, click here.
B. Can we prove the equality?
Start by constructing a line though A parallel to BC. Now we have four sets of similar triangles, highlighted as follows:
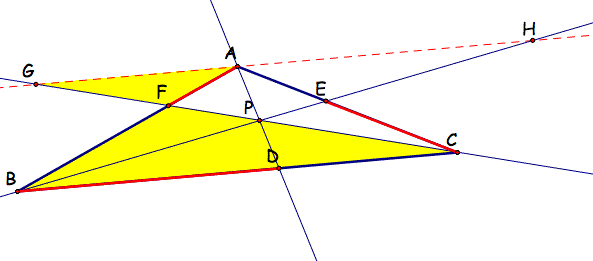
So, 1) BC/AG = BF/AF = CF/FG;

2) AG/CD = AP/DP = GP/CP;
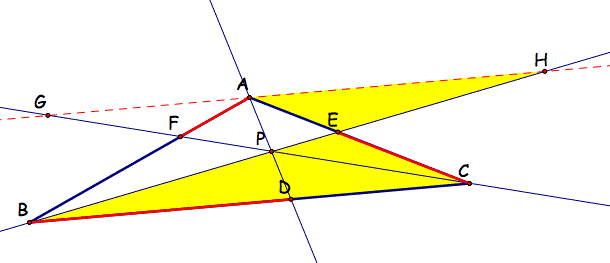
3) AE/CE = AH/BC = EH/BE; and
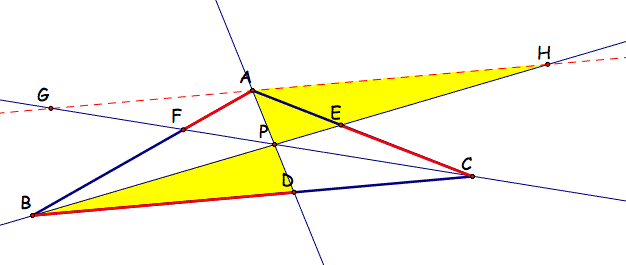
4) AH/BD = AP/DP = HP/BP.
From 2) AG/CD = AP/DP and 4) AH/BD = AP/DP, so AG/CD = AH/BD.
Now AG/CD * BC/AG * AH/BC = AH/BD * BF/AF * AE/CE.
So, AH/CD = AH/BD * BF/AF * AE/CE.
and 1 = (CD * BF * AE) / (BD * AF * CE)
therefore (CD * BF * AE) = (BD * AF * CE). QED
C. Show that when P is inside triangle ABC, the ratio of the areas of triangle ABC and triangle DEF is always greater than or equal to 4.
Here is a picture:
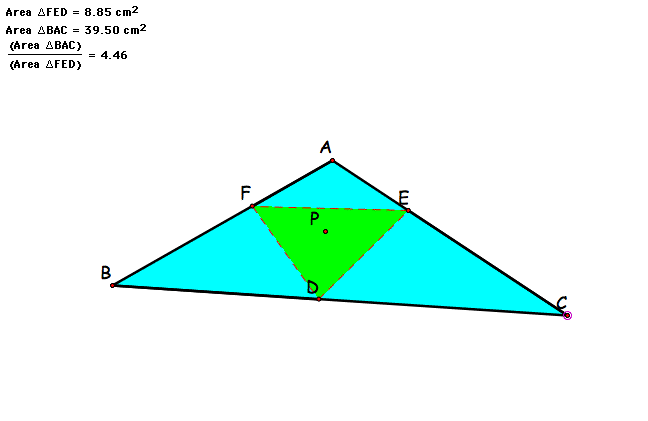
You can manipulate the GSP sketch by clicking here.
When is the ratio equal to 4? By manipulation,
it appears to be equal to 4 when D, E, and F are at the midoints
of their segments (or, to put it another way, when P is the centroid
of triangle ABC).