

Graphing Calculator 3.2 and xFunction are suggested for these investigations.
Some of them could be done with a TI-83 or similar.
Polar Coordinates are a way to describe the location of a point on a plane. A point is given by coordinates (r,theta). r is the distance from the point to the origin, and theta is the angle measured counterclockwise from the polar axis to the segment connecting the point to the origin.
Investigate
When a and b are equal,
and k is an integer, this is one textbook version of the "
n-leaf rose."
Compare with
for various k. What if . . . cos( ) is replaced with sin( )?
Let's look at r=a+bcos(ktheta) first.
This is the graph of a=1, b=1, k=1.

a=2, b=1, k=1
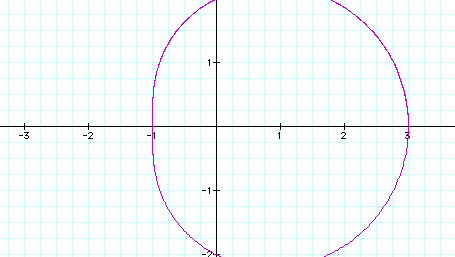
a=1, b=2, k=1
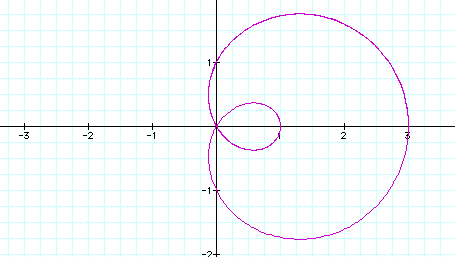
a=1, b=1, k=2

a=1, b=1, k=5

a=1, b=1, k=10
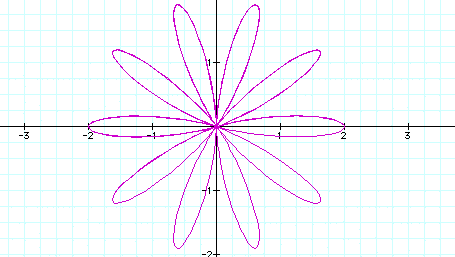
Now let us investigate our equation
for different values of b and k. First look at b=1 and various values for k.
k=1, k=2
k=3, k=5
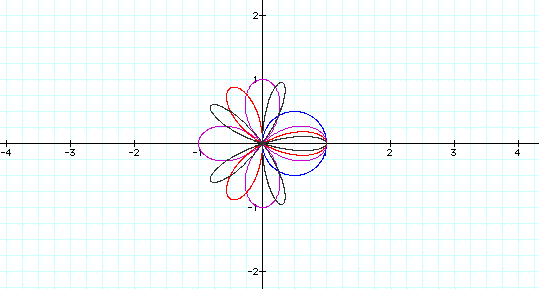
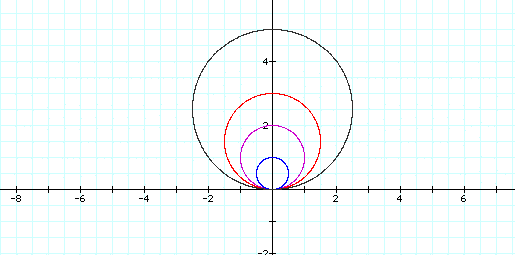
Now let us look at various values for b when k remains equal to 1. Notice that larger values for b produce larger circles.
b=1, b=2
b=3, b=5

Now what if we replace cos with sin in our second equation? Let's see what happens.
b=1, b=2
b=3, b=5
Return to Home Page