
Assignment 9
Pedal Triangles
by Jeff Hall
Problem 1a
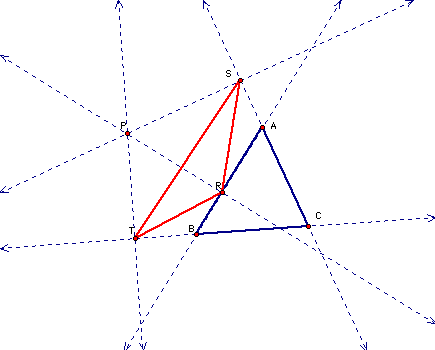
Draw any Triangle ABC. Point P is any point in the
plane. Construct the perpindiculars to the sides
of ABC (extended if necessary) and label the three
intersections R, S, and T.
This Triangle RST is called the Pedal Triangle for
Pedal Point P.
Problem 1b
A pedal triangle can be formed from any triangle where
P is any point in the plane of ABC.
A GSP tool, titled PedalTriangle,
has been created to assist in the creation of these triangles.
As in Assignment 5, click on the link above and choose
the Pedal Triangle tool to use it.
Problem 2
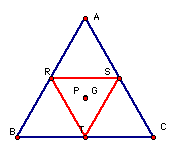
What happens when P is on the centroid of the triangle?
You get this:
 Notice that the pedal triangle is similar to a medial
triangle,
except that the vertices of are not on the midpoints
of Triangle ABC.
If Triangle ABC is an equilateral triangle, then Triangle
RST becomes
a medial triangle.
Notice that the pedal triangle is similar to a medial
triangle,
except that the vertices of are not on the midpoints
of Triangle ABC.
If Triangle ABC is an equilateral triangle, then Triangle
RST becomes
a medial triangle.
Problem 3
Now what happens when the Point P is on the incenter
of Triangle ABC?
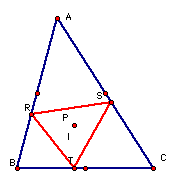 Again, it created a triangle similar to the medial
triangle of Triangle ABC.
Since the Incenter and Centroid are the same point
in an equilateral triangle,
the Pedal Triangle is a medial triangle when P is on
I.
Again, it created a triangle similar to the medial
triangle of Triangle ABC.
Since the Incenter and Centroid are the same point
in an equilateral triangle,
the Pedal Triangle is a medial triangle when P is on
I.
Problem 4
What if P is on the Orthocenter?
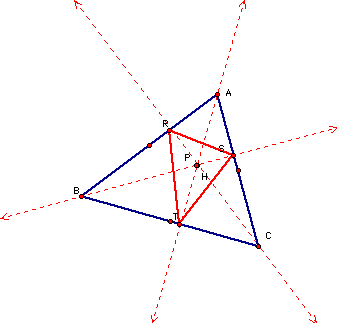 As you can see from the rays, the pedal triangle is
the orthic triangle when P is on H.
But what happens when H is outside the lines of Triangle
ABC?
As you can see from the rays, the pedal triangle is
the orthic triangle when P is on H.
But what happens when H is outside the lines of Triangle
ABC?
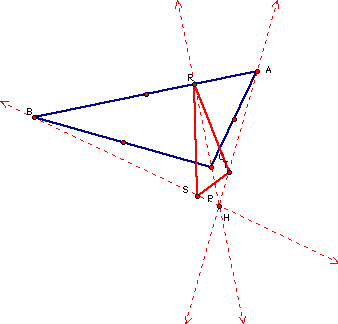 The pedal triangle becomes the orthic triangle of Triangle
HBC.
The pedal triangle becomes the orthic triangle of Triangle
HBC.
Problem 5
What if P is on the Circumcenter?
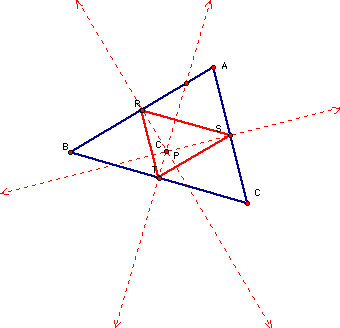 The pedal triangle is now the medial triangle for Triangles
ABC...and it's not equilateral!
But what happens when the Circumcenter lies outside
of Triangle ABC?
The pedal triangle is now the medial triangle for Triangles
ABC...and it's not equilateral!
But what happens when the Circumcenter lies outside
of Triangle ABC?
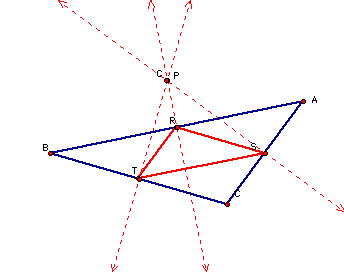 The pedal triangle remains the medial triangle despite
the circumcenter lying outside.
The pedal triangle remains the medial triangle despite
the circumcenter lying outside.
Problem 7
What if P is on a side of the triangle?
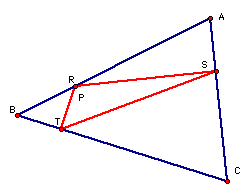 Is Triangle RST always contained in Triangle ABC when
P is on a side?
Is Triangle RST always contained in Triangle ABC when
P is on a side?
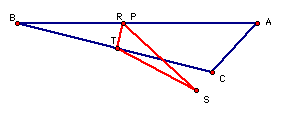 As you can see, the pedal triangle does not have to
be contained in Triangle ABC.
As you can see, the pedal triangle does not have to
be contained in Triangle ABC.
Problem 8
What if P is on one of the vertices of Triangle ABC?
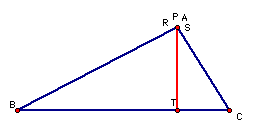 The pedal triangle forms the altitude from the vertice
to the corresponding base.
The pedal triangle forms the altitude from the vertice
to the corresponding base.
Problem 9
As we saw in Problem 8, when P is on one of the vertices,
the pedal triangle becomes a straight line.
Thus, it becomes a degenerate triangle, called the
Simson Line.
There are multiple conditions that can be met in order
to creat the Simson Line.
Placing the Point P on any of the three vertices of
Triangle ABC creates the line.
Placing Point P on the circumcircle of Triangle ABC
will also create the Simson Line.
Problem 10 and 11
Construct the circumcircle of Triangle ABC. Now create
a larger circle around the same center point C.
Animate Point P around this circle while tracing the
midpoints of Triangle RST. What do you see?
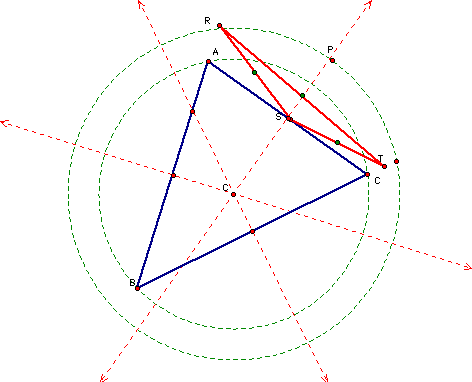 Here is the construction you get for Problem 10. Click
here to see Point P animated
around the circle and the resultant traces of the midpoints.
You may click the "Move Point" button
to move the path of Point P onto the circumcircle of
Triangle ABC.
It is interesting to note that the traces create ellipses
distinct from each other. These ellipses
originally move around the vertices of Triangle ABC,
but as the path of Point P converges onto
the circumcenter, the ellipses pass through each vertex.
Here is the construction you get for Problem 10. Click
here to see Point P animated
around the circle and the resultant traces of the midpoints.
You may click the "Move Point" button
to move the path of Point P onto the circumcircle of
Triangle ABC.
It is interesting to note that the traces create ellipses
distinct from each other. These ellipses
originally move around the vertices of Triangle ABC,
but as the path of Point P converges onto
the circumcenter, the ellipses pass through each vertex.
Problem
11a
Construct lines on the sides of the Pedal Triangle
and trace them as P moves around in a circular path.
Click here
to see the traces.
Notice that the traces look to create a triangular
figure similar to Triangle ABC.
Problem 11b
Moving Point P onto the circumcircle creates a similar
but smaller figure.
Note that this illustrates the envelope of the Simson
Line as described in Problem 9.
Problem 12
Repeat this process with the P travelling around a
circle centered on C
but with a smaller radius than the circumcircle.
Click here
to see what happens.
Problem 13
Is there a point on the circumcircle for P that has
side AC as its Simson line? AB? BC?
For this solution, I created diameter lines of the
circumcircle from each vertex.
I labeled these points A', B', and C'.
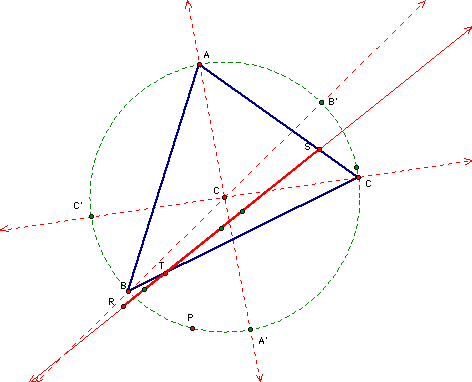 Click here
to move Point P to each of these new points.
As you can see, when P is on one of the prime points,
the pedal triangle
has one of the sides of Triangle ABC as its Simson
Line.
Click here
to move Point P to each of these new points.
As you can see, when P is on one of the prime points,
the pedal triangle
has one of the sides of Triangle ABC as its Simson
Line.
Problem 14
Now, connect Point P on the circumcircle to the orthcocenter
of Triangle ABC. Like so:
 How do the Simson Line and Segment PH intersect? Click
here to watch move P
around the circle.
As you can see, the two segments overlap when P is
on the vertices of Triangle ABC.
This is to be expected since we saw that the Simson
Line was an altitude of Triangle ABC in Problem 8.
How do the Simson Line and Segment PH intersect? Click
here to watch move P
around the circle.
As you can see, the two segments overlap when P is
on the vertices of Triangle ABC.
This is to be expected since we saw that the Simson
Line was an altitude of Triangle ABC in Problem 8.
Problem
15
Select two pedal points on the circumcircle and construct
their Simson lines.
Compare the angle of intersection of the two Simson
lines with the angular measure
of the arc between the two pedal points.
Here is my construction:
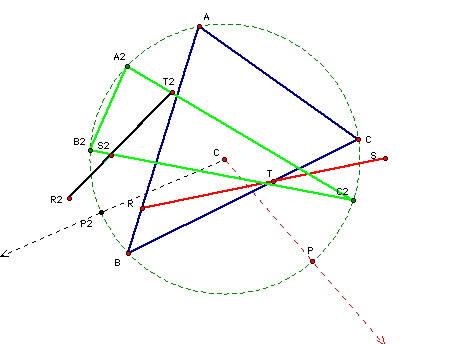 The new Pedal Point is labeled P2, and was created
from Triangle A2, B2, C2.
The Pedal Triangle, currently in the form of Simson
Line, are formed by the points R2, S2, T2.
Click here to manipulate
the Pedal Points.
The new Pedal Point is labeled P2, and was created
from Triangle A2, B2, C2.
The Pedal Triangle, currently in the form of Simson
Line, are formed by the points R2, S2, T2.
Click here to manipulate
the Pedal Points.
Problem 16
Animate the Pedal point P about the incircle of ABC.
Trace the loci of the midpoints of the sides. What
curves result?
First, here is the construction:
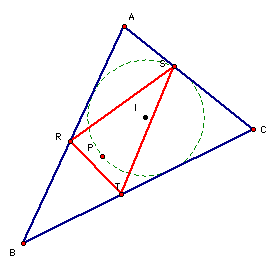 Click here
to animate P around the incircle.
After one revolution, the traces will look like this:
Click here
to animate P around the incircle.
After one revolution, the traces will look like this:
 Now, repeat if ABC is a right triangle. Here is what
it looks like:
Now, repeat if ABC is a right triangle. Here is what
it looks like:
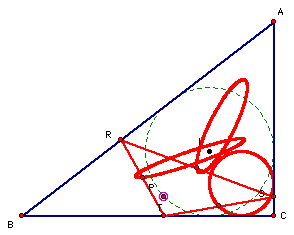 Notice that one of the midpoint traces creates a circle
tangent to AC and BC.
Notice that one of the midpoint traces creates a circle
tangent to AC and BC.
Problem 17
Construct an excircle of triangle ABC. Animate the
Pedal point P about the excircle
and trace the loci of the midpoints of the sides of
the Pedal triangle. What curves result?
Look at the angle bisectors through the center of the
excircle. How are the loci positioned
with respect to the angle bisectors?
Click here
to animate P about an excircle of Triangle ABC.
Here are the traces of the midpoints. As you can see,
they create ellipses, all of which cross
over the angle bisector. Notice that one ellipse is
split evenly by the bisector.

Return




















