

Graphs in the xb plane.
Case 1: C = 1
First, let's look at the equation
If we graph this in the xb plane, we get the following graph.

This is the graph of the equation ![]()
To graph a value of b on the graph we add a line parallel to the x-axis. If this line intersects the graph in the xb plane the points of intersection are the roots of the original equation. Here the graph with that line added.
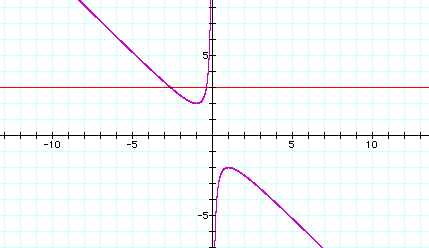
From looking at the graph we can see that there are two positive roots if b>2 and two negative roots if b<-2. We can also see that there is one positive root if b=2 and one negative root if b = -2. There are no roots if -2< b < 2.
Case 2: c = -1
Now we will look at the equation
When we graph this in the xb plane we get this graph:
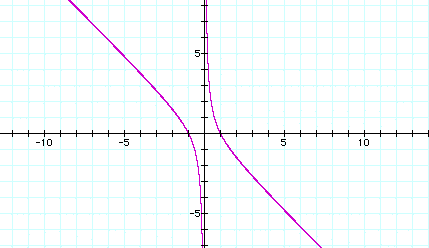
This is the graph of the equation ![]()
To graph a value of b on the graph we add a line parallel to the x-axis. If this line intersects the graph in the xb plane the points of intersection are the roots of the original equation. Here the graph with that line added.
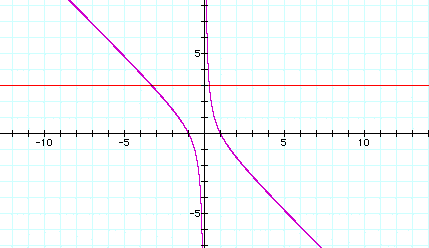
Looking at this graph we can see that this equation has two roots at all times. The equation has two positive roots if b > 0 and two negative roots if b < 0.
Case 3: Other values of c
Now, we will look at other values of c. Here is a graph of the same equation in the xb plane with c = -10. -5. 0, 5, 10.
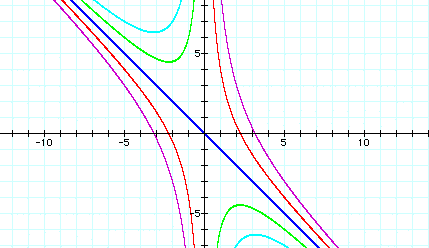
Looking at the graph we notice when c is negative (the purple and red graphs) there will always be two roots. We also notice when c is positive (the green and teal graphs) there are multiple things that can happen. When c is positive there can be two positive roots, one positive root, one negative root, two negative roots, or no roots. We also notice that when c = 0 the graph becomes linear (the blue graph). This makes sense because when that equation is simplified we get y = -x which is the line that is shown above.