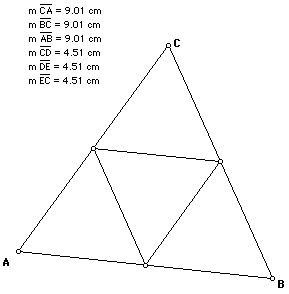
If the original triangle is equilateral, then the triangle of median is equilateral. Will an isosceles original triangle of medians? Will a right triangle always generate a right triangle of medians? Under what conditions will the original triangle and the medians triangle both be right triangles?
First let's look at an equilateral triangle.
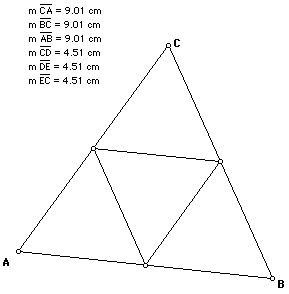
As you can see, that triangle of medians is also equilateral. And we know from previous Geometry classes that the sides in the smaller triangle are half the length of the original triangle's sides.
Now let's look at the isosceles triangle.
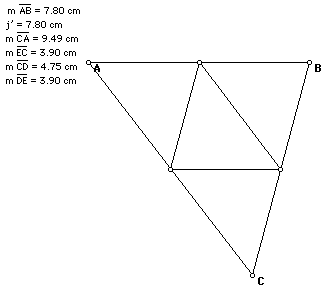
This triangle of medians is also an isosceles triangle, where the two sides of the original triangle that are equal are opposite the two sides that are equal in the triangle of medians.
Now let's look at a right triangle. Before we look, I feel that the triangle of medians will be a right triangle and the right angle in the triangle of medians will be opposite the right angle in the original triangle.
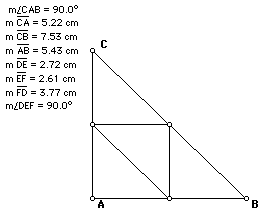
As we see I was right. So what conjectures can I make? Well first of all inall of the cases we have seen, the triangle of medians is a similar triangle. The sides opposite the sides in the original triangles are half the length and the angles are the same. Therefore the ratio is 2 to 1 for the original triangle and the triangle of medians.