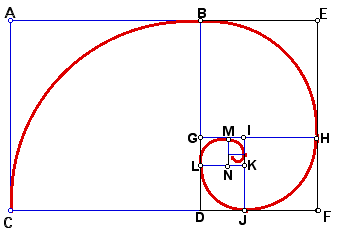
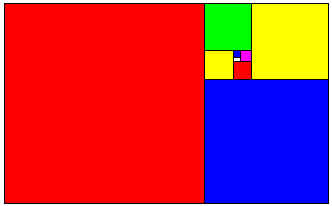
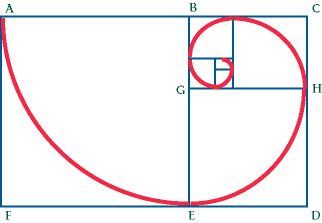
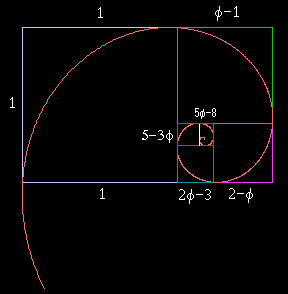
Given a rectangle having
sides in the ratio 
, the golden ratio
is defined such that partitioning the original
rectangle into a square and new rectangle results in a new rectangle
having sides with a ratio . The
successive dividing a golden rectangle into squares lie on a logarithmic
spiral. If the top left corner of the original square is positioned
at (0,0), the center of the spiral occurs at the position
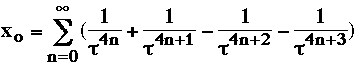
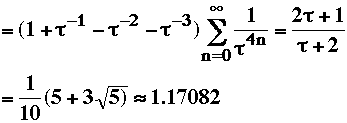
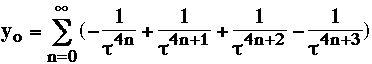
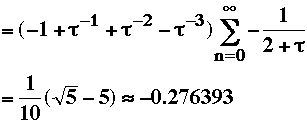
and the parameters of the
spiral 
are given by
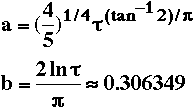
(http://mathworld.wolfram.com/Golden.Triangle.html)