The problem is that given three segments that represent the medians of a triangle, construct the original triangle using straightedge and compass.

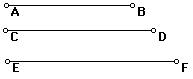
Given the three segments above as medians of a triangle, first construct the triangle of the medians. See the figure below. I have changed the colors of the segments so that we can more easily identify them. Median AB is now blue, median CD is red and median EF is green. Assume the green median is in its proper position. Let F be the end of the median at the vertex of the triangle. Find the midpoint of the red median and construct a ray from F through the midpoint. See below.

Now trisect the green and red medians.

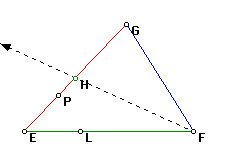
Now construct a line parallel to the red median through the point L.

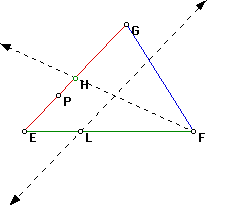
Now mark off a segment on the parallel line the same length as the red median such that one end of the segment is on the ray extending from point F.

This segment is now in place as another median of the triangle. Point U is the midpoint of the side that lies along the ray from point F. Point V is another vertex of the triangle, and now point E is the midpoint of the third side of the triangle. Draw a ray from point V through point E. The intersection with the ray from point F is the third verttex. Draw segments connecting the vertices and the triangle is completed.

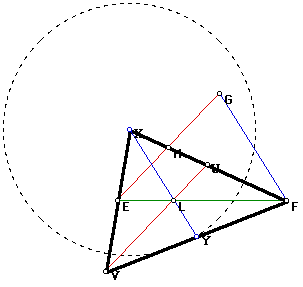
The completed triangle appears above. To do a final check, draw a segment the same lenght as the blue median from the point X through the point L and check that it is a median

 If you want to see a Geometer's Sketch Pad script that constructs a triangle from its medians, click here.

Return