Ancient Greek mathematicians interested in nonmetrical geometry were enthralled with three leading problems of the time: the quadrature of the circle, the duplication of the cube and the trisection of any given angle. To make matters more interesting, one was only allowed to use an unmarked straightedge and a compass. This was because, "for aesthetic reasons, the straightedge and circle were considered to be the only perfect curves" (Calinger, 42). It is also evident from their symmetrically designed cities and geometric pottery that the Greeks were a people who "preferred a natural visual approach to the natural world and stressed order" (Calinger 38). By some at the time, it was considered a foundational crisis when Theodorus of Cyrene and his pupil Taetetus discovered that most of the square roots from the square root of three to the square root of 17 are irrational (incommensurable with a unit, they would say). If incommensurable numbers caused an upheaval, it is no surprise that the Greeks sought order in the midst of chaos. This is shown again as they sought the quadrature of various objects. Quadrature of a plane figure is the construction of a square having area equal to that of the original plane figure. for those who sought order, "there was much appeal in the process of replacing the asymmetric by the symmetric, the imperfect by the perfect, the irrational by the rational" (Journey Through Genius, pg. 12).
In Hippocrates' day (460-380 B.C.), the quadrature of irregular polygons had been accomplished. Though this was respected, it was overshadowed by the fact that these were rectilinear objects. The more challenging issue was whether or not curvilinear figures were able to be squared (Journey Through Genius, pg. 17). Through Hippocrates did not square the circle, he did successfully square the lunule, from which he falsely believed the quadrature of the circle could be derived. Hippocrates methods for squaring the lunule--a plane figure bounded by two circular arcs (a crescent)--is on what I will focus. His argument relied on three preliminary results: (1) The Pythagorean theorem (2) an angle inscribed in a semicircle is right (3) the areas of two circles or semicircles are to each other as the squares of their diameters.
Though Pythagorus was able to state his famous theorem relating the hypotenuse and legs of right triangles, he was probably not able to prove it. Euclid, however, records the theorem's proof in Book VII of his Elements. It is believed that Thales of MIletus, a contemporary of Hippocrates, successfully proved that (1) an angle inscribed in a semicrcle is right (2) the base angles of an isosceles triangle are equal in measure and (3) the sum of the angles of a triangle is equivalent to two right angles. Though there are no records of his proofs, it is believed by some that it is Thales' work recorded in Book III of Euclid's Elements. We will take a quick look at the proof that an angle inscribed in a semicircle is right.
Construct a semicircle with center O and diameter AC. Let B be any point on the semicircle. Construct the segment AO.
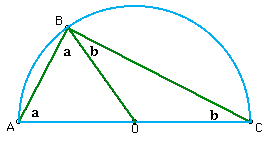
We must show that angle BAC is right. Since segments AO and OB are both radii of semicircle ABc, they are equal in length. Therefore, the triangle ABO is isosceles. Similarly, triangle OBC is isosceles. Therefore, because the base angles of an isosceles triangle are equal in measure, the measure of angle ABO is equal to the measure of angle OAB. Let angle AbO = angle OAB = a. similarly the measure of angle COB is equal to the measure of angle OBC. Let angle COB = angle OBC = b. Therefore, the following is true:
=
measure of (angle OAB) + measure of (angle ABC) + measure of (angle
BCO).
=
a + (a+b) = 2a + 2b = 2(a+b) . Thus,
=
(a+b). Thus, angle ABC is right. Thus, any angle inscribed in
a semicircle is right.
We learn about Hippocrates' methods through the writings of SImplicius. It is now known that there are five types of lunules that can be squared. The type of lune that HIppocratesset out to square was one whose circumference was a sermicircle. Let's begin witht the following semicircle with center O and radii AO =OB.
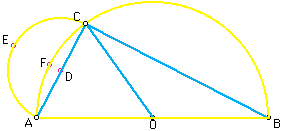
Construct the perpindicular segment through C, a point on the semicircle, and O. We can now construct segments AC and CB. As was knowon to Hippocrates, the inscribed triangle ACB is a right triangle. Angle ACB is right because it is inscribed in the semicircle. Because the length of segment CO is equivalent to itself, segment A) = OB and the measure of both angle AOC and angle COB is ninety degrees, triangle AOC is congruent to triangle COB. Therefore, side AC is congruentt o side BC. Because AO and OC are both radii of the semicircle, the length of AO is equivalent to the length of OC. Therefore, triangle AOC is an isosceles right triangle. Now, construct themidpoint of the segment AC. Call themidpoint D. Using D as the center and Ad as the radius, construct the semicircle AEC. Let F be a point on the semicircle ACB, such that F is between A and C and let E be a point on our newly constructed semicircle with base AC. Therefore, we define the lune AECF as the region between the arc AEC and the arc AFC. Applying the Pythagorean theorem, we see that the segments AB, AC and BC have the following relationship:
.We can now apply the principle relating the area of two semicircles and the squares of their diameters. As previously stated, it had been shown before HIppocrates that the ratio of the areas of two semicircles is equivalent to the ratio of their squares of their diameters. Applying this principle to our semicircles, we see that:
Because (Length of AB) = 2(Length of AC), we get the following:
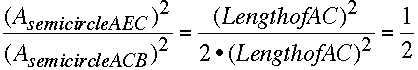
This tells us that semicircle AEC has half of the area as semicircle ACB. Well, it is also true that sector AFCO has half of the area as the semicircle ACB. Therefore, the area of semircircle AEC is equivalent to the area of sector AFCO. One can see that the sector AFCO and the semicircle AEC share the region AFCD. Therefore, if we subtract off the overlapping region, then the area of the lune AECF should be equivalent tot he area of the triangle ACO.
Therefore, 
. As previously noted, mathematicians had
already succeeded in squaring polygonal figures, such as the triangle.
Hippocrates then knew that if the lune could be shown to have
the same area as a triangle, then the lune could be shown to have
the same area of a square.
Hippocrates' work, described above, was completed with accuracy and triumph. His extensions of this work into the quadrature of the circle, however, were found flawed. Thel ine of reasoning is not given rigorously, but is outlined as follows:
Construct a circle with center O and radius Cd such that CD = 2AB. Within the larger circle, construct a regular hexagon with each side equivalent to the radius, C) = OD. Call this regular hexagon CEFDGH. Now, as described earlier, construct a lune upon each side of the hexagon (see figure below).
We decompose thehexagon-lune configuration in two ways. One way is as a regular hexagon plus six semicircles. for the second decomposition, remember that eac side of the hexagon is equivalent in length to CO which is equivalent to the length of AB.Therefore, the sum of the areas of the six semicircles is equivalent to the sum of the areas of three of the small circles. Thus, the second decomposition is the same as the large circle plus six lunes. With these two decompositions, the following relationships are true:
Because the diameter of the large circle is twice that of the small, we get the following relationship:
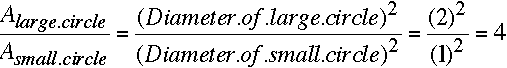
So, the area of the large circle is four times that of the small circle. Returing to our previous equation, we get:
Thus, since the hexagon an be squared and the six lunes can each be squared, the circle can be squared. This argument flows quite smoothly,however, there is one crucial error. Though Hippocrates, as shown earlier, was able to square a lune based on a square inscribed inside of a circle, he had not shown it possible to square a lune based on a regular hexagon inscribed in a circle. To Hippocrates' credit, it is doubted by some historians that this flaw is his. Rather, it is likely that his proofs were confused by commentators such as Simplicius. This is not the only flaw in the idea that the circle can be squared. However, the impossibility of the quadrature of the circle would not be proven rigorously until 1882, by Ferdinand Lindemann.
This discussion raises the following question: what are the five lunes that are said to exist. Hippocrates mistakenly thought that the lune based on a regular hexagon inscribed in a circle was the same as a lune based on a square inscribed inside of a circle. We have been told that these are different and so we know two of the five lunes that exist. What are the other three?