WHAT MAKES A QUADRATIC TICK?!?

BY DIXIE WILLIFORD

In the following pages, I will explore how certain manipulations to a quadratic function alter the graph.

The quadratic function will be working with is: y = 2(x^2) + 3x- 4.

.

 

Here we have the graph of our quadratic equation, y. The parabola has its vertex at (-.75,-5.125). We also can observe that the parabola is concave up. Let us see what happens if we replace every x with (x-4).

Our new equation will be : .

y = 2(x^2)+3x-4 and y = 2((x-4)^2 )+3(x-4)-4

 

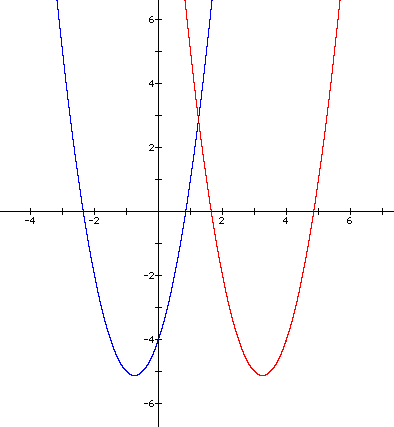
It is obvious, by looking at the graph, that replacing x by (x-4) shifted the vertex of the original parabola to the right. What is the vertex of our new equation and how does it relate to the vertex of our original equation? Well, the general form or "vertex form" of a quadratic equation is:

, where (h,k) is the vertex of the parabola.

Therefore, we must complete the square in order to obtain this general form of the equation and to discover the changes that have occurred. First, let us complete the square of the equation:

y =2(x^2)+3x-4

2(x^2) + 3x - 4 = 0

2 [{(x^2) +(3/2)x +(9/16)} - 2 - (9/16)] = 0

2((x+(3/4))^2) - (41/8) =0

By looking at the "vertex form" of the equation, we see that the vertex is at ((-3/4),(-41/8)).

Now let us complete the square of the equation: y = 2((x-4)^2) +3(x-4)-4. Again, this will again tell us the vertex of the equation.

2((x-4)^2) +3(x-4)-4 = 0

Simplifying, we get: 2(x^2) -13x+16 = 0

Now, we begin to complete the square:

2[{(x^2) + (13/2)x + (169/16)} +8 - (169/16)] = 0

2((x-(13/4))^2) - (41/8) = 0.

This "vertex form" of our equation tells us that the vertex lies at ((13/4),(-41/8)).

Therefore, replacing x by (x-4) in our original equation: y = 2(x^2)+3x-4, shifted the graph to the right by

(3/4) + (13/4) = (16/4) = 4 units.

The original parabola has its vertex in the fourth quadrant, very near the y-axis. Shifting the graph 4 units to the right, therefore, moved its vertex into the fourth quadrant. How could we change the original equation in order to move the parabola's vertex into the second quadrant? Let us gather our facts in order to consider this question.

OBSERVATIONS:

1. is the vertex form of the equation of a quadratic function,where h is the x-coordinate of the vertex and k is the y-coordinate of the vertex.

2. The original equation, in its vertex form, is . Changing h from (-.75) to (13/4) is what caused the shift of the vertex into the fourth quadrant. k=(-41/8) stayed the same and the parabola did not move vertically at all.

 

Therefore, in order to move the original parabola, ,into the second quadrant, let us alter the value of k and let h remain constant. This will shift the parabola directly upward from the fourth quadrant. Let k=6.

Therefore,.

The expanded form of our new equation is:

Let us now look at the picture of this equation:

 

 

You can see that the vertex of our new parabola does indeed lie in the second quadrant at (-.75,6). Our manipulation was successful.

Let's try one last manipulation. How could we change our original equation, , in order to create a parabola that is concave down and that shares its vertex with the original parabola.

Well, so far, we have only moved our original parabola left-right and up-down. We have not changed its direction of concavity. Also, we have not changed the value of "a" in the general equation:. Could this be the missing link? Let's try letting a=-2 rather than a=2. Because we are convinced that h and k, in the vertex form of the parabola's equation, determine the vertex, we will let them remain the same as well. Let 's take a look at the graph we obtain with our manipulated equation, .The expanded form of this equation is:.

and

As you can see, , does indeed share its vertex with and is concave down as well. Our predictions proved to be true!

In conclusion, in order to manipulate a quadratic curve, it is best to first manipulate the quadratic equation associated with that curve into the vertex form of the equation. Once you have obtained the vertex form of the equation, ,you can alter a, h and k in order to place and reorient the curve.

RETURN