Note: I recommend that this page be printed out, so that the instructions are easier to follow.
In order to successfully complete a proof,
it is important to think of the definition and the construction
of a parallelogram.
In the following outline, I will provide the statements, you
provide the reasons.
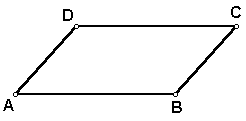
Prove: *If a quadrilateral is a parallelogram, then the consecutive angles are supplementary.
Given: Parallelogram ABCD

Prove: Angle BAD and angle CBA are supplementary. *This is a proof for a single pair of consecutive angles. To fully prove the statement above, the 3 other pairs of consecutive angles must also be proved to be supplementary.
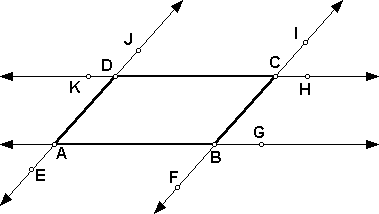
Proof:
Extension:
If you have any questions while trying to complete this investigation, or suggestions that would be useful, especially for use at the high school level, please send e-mail to esiwdivad@yahoo.com.
Return to the Table of Contents.