Department of Mathematics Education
J. Wilson, EMAT 6680

For more information on GSP, contact the publisher, Key Curriculum Press. To find instructions on setting up GSP as a helper application click here.

This investigation begins with the construction of a pedal triangle and focuses on the discovery of properties of the pedal triangle when the pedal point is confined to the centroid, incenter, orthocenter, or circumcenter. Centers of triangles are a standard topic in high school geometry courses. Pedal triangle investigations provide interesting extension problems.

I have provided only discoveries. This investigation could easily be adapted for students to follow the same discovery path, or could be altered to investigate one topic with more depth. Students should be able to complete proofs of, or pertaining to relationships discovered within this investigation. In addition, I have only scratch the surface of discoveries based on pedal triangles. For example, when the three vertices of the pedal triangle are collinear (the pedal triangle is degenerate), the line segment is called the Simson Line. The inspiration for this investigation, which suggests many more investigations pertaining to pedal triangles, was developed by Dr. Wilson. Click here to link to his other suggestions for investigations of pedal triangles and the Simson Line.

I. Constructing the Pedal Triangle

• Construct any triangle ABC by first using line constructions and then constructing the segments of the triangle.
• Construct any point P.
• Construct the perpendiculars from point P to the sides of triangle ABC. Note: Construct the perpendiculars using the lines of triangle ABC.
• Construct the intersection points of the perpendiculars to the sides of triangle ABC. Label the points R, S, and T.
• Construct the triangle RST.
• Triangle RST is the Pedal Triangle to triangle ABC, where P is the Pedal Point.
• Click here for the script.
• Click here for the sketch.

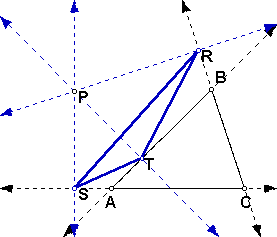
II. The pedal point and the centriod of triangle ABC are concurrent.

Findings:

• The pedal point P is always located in the interior of the pedal triangle RST.

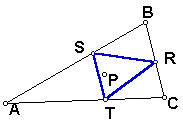
• The vertices of the pedal triangle remain on the sides of triangle ABC, until triangle ABCbecomes significantly obtuse.

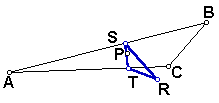
• When the three vertices of triangle ABC are collinear (triangle ABC is a degenerate triangle), the vertices of the pedal triangle RST and the pedal point P are concurrent.

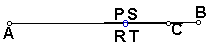
• When the three vertices of triangle ABC are collinear and two of the vertices are concurrent, two of the vertices of the pedal triangle RST and the pedal point P are concurrent and the third vertex of the pedal triangle RST is concurrent to the two concurrent vertices of triangle ABC. In addition, the degenerate triangle ABC is divided by the pedal point P (and the concurrent points to P) into two segments in which the longer segment is twice the distance as the shorter segment.

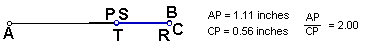
To investigate further, click here for the sketch.

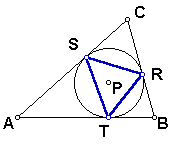
III. The pedal point and the incenter of triangle ABC are concurrent.

Findings:

• The vertices of the pedal triangle RST are always the points of tangency of the inscribed circle of triangle ABC.

To investigate further, click here for the sketch.

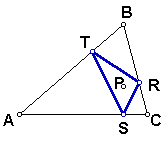
IV. The pedal point and the orthocenter of triangle ABC are concurrent.

Findings:

• When triangle ABC is an acute triangle, the pedal triangle RST is also the orthic triangle of triangle ABC.

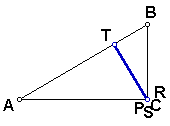
• When triangle ABC is a right triangle, the vertices of the pedal triangle RST are collinear (triangle RST is a degenerate triangle) with the pedal point P and two of the vertices of the pedal triangle RST concurrent to the vertex of the right angle of triangle ABC. In addition, the segment RST is the altitude of triangle ABC from the vertex of the right angle perpendicular to the opposite side.

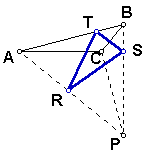
• When triangle ABC is an obtuse triangle, the pedal point P is outside triangle ABC. If segments are constructed from the pedal point to the two vertices of the acute angles of triangle ABC, a triangle is constructed in which the pedal triangle RST is the orthic triangle of the constructed triangle. As pictured, the pedal triangle RST is the orthic triangle of triangle ABP.

To investigate further, click here for the sketch.

V. The petal point and circumcenter of triangle ABC are concurrent.

Findings:

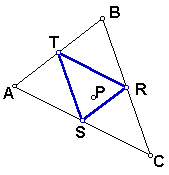
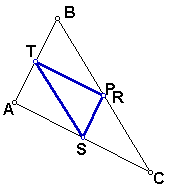
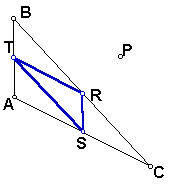
• For any triangle ABC, the pedal triangle RST is also the medial triangle of triangle ABC.

To investigate further, click here for the sketch.

If you have any comments concerning this investigation that would be useful, especially for use at the high school level, please send e-mail to esiwdivad@yahoo.com.

Return to my homepage.