Click to check Practice Problems from
Radical Review and the Geometric Mean
Recall: The Geometric Mean, x, between two positive numbers a and b is
Recall: the parts of a right triangle:
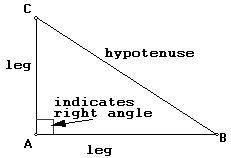
Activity,Create a right triangle:
The following GSP sketch will lead you through the steps to construct a right triangle When done highlight the triangle and copy it to your own sketch and save as right1.gsp.
After pasting the triangle into your sketch, label the vertices as in the triangle above. Label the hypotenuse.
Drag the vertices around, by construction the right angle is preserved.
Activity, Similar Triangles
In GSP, open your right1.gsp and highlight point A and segment CB, construct a perpendicular line. Create the altitude AD and hide the construction line. Construct Polygon ABC and ADC.
What can you say about the three triangles ABC,ADC and ADB ? Save this sketch as right2.gsp.
Print it out and put in your folder.
Activity1: Open Right2.gsp in GSP
1. Click on D and Mark as center in the transform menu.
2. Click on Triangle ADC and then under the the transform menu, select rotate. Rotate by 90 degrees. What conjecture can you make about triangles ADC and ADB ? Print out the resultant picture for your presentation.
Activity2: Open Right2.gsp in GSP
1. Click on segment AC and Mark as Mirror in the transform menu.
2. Choose triangle ADC and select reflect in the transform menu.
3. Mark C as center in the transform menu.
4. Click on the reflected triangle and choose rotate in the transform menu. Click on the measure of angle DAB or DCA and then OK Change the color of the triangle to a color not present in the sketch in the display menu..
5. What conjecture can you make about triangles ABC and ADC?
Recall: Similarity of triangles is transitive. What does that tell us about triangles ABC and ADB?
Similarity Theorem: The altitude drawn from the vertex of the right angle of a right triangle to its hypotenuse creates two triangles, both of which are similar to the original triangle and each other.
Click the lightbulb to practice what you have learned.