

Problem 6: Graph
i. Overlay a new graph replacing each x by (x-4).
ii. Change the equation to move the graph into the second quadrant.
iii. Change the equation to produce a graph concave down that shares the same vertex.
I didn't like graphing much when I was in school many years ago, therefore I didn't pay much attention when this topic was discussed. Any of these graphing problems are excellent reviews for me.
The first thing I did with this problem was to graph the original equation.

At this point I couldn't tell you exactly what had just happened, but when I applied "i" to this graph, it began to make a little more sense. I replaced each x by (x-4).

By replacing the x with (x-4), the graph was moved 4 units to the right. It did not change it's shape or move in any other direction. I haven't quite figured out why it moved to the right 4 units.
Now I need to try to move the graph into the second quadrant, which is the top left hand corner of the graph. If (x-4) moved the graph 4 units to the right, maybe (x+4) will move the original graph 4 units to the left.


I was correct. Now I need to move the blue graph up into the third quadrant. My guess would be that the (-4) should be changed to a positive number and the graph will move up. Since the original graph crosses the y axis at -5, I am going to try using (+6).


The graph did move into the 2nd quadrant. Now I will delete the first 3 graphs and just work with the one in the 2nd quadrant. My next objective is to change the equation to produce a graph concave down that shares the same vertex. I will attempt this by trial and error and then see if I can make a general statement on how to find the equation with an easier method.


Something from my past told me that if I negated the squared part of the problem, that it would "flip" the parabola. So that's what I did and it worked. I just thought it would flip it at its lowest point, but it didn't. Now I will try to move the green parabola down and to the left to share the same vertex.


I tried 2 other graphs before I ended up with this blue one. I knew that (+6) moved the parabola "up" from where it began. I needed it to move down, I changed the (+6) to (+5) and then (+4). Neither graph was low enough to be anywhere close to the vertex of the red parabola. So my final choice was (+3 1/2). I will probably have to adjust it again later, but this is a good place for me to start. Next, I need to move the blue graph to the left. I know I will probably need another negative somewhere, so I will try a few graphs.


I made a very obvious guess. I knew that the "c" part of the equation moved the graph up and down and I knew that the "a" part of the equation "flipped" the parabola. So I began with the "b" part of the equation. I changed (+3) to a (-3). The parabola moved to the left directly underneath the red graph with which I want it to share a vertex. The only thing left to do is to move the brown up slightly so that they touch. To do this I must change the 7/2 ever so slightly.


I chose a fraction half way between 4 and 3 1/2 and ended up with 3 3/4. That seemed to do the trick.

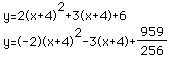
The above is a graph showing the 2 parabolas sharing a vertex. I came up with that "absurd" fraction by trial and error. I would double the fraction and then subtract "1" from the numerator until the green parabola moved down just enough to appear to share a vertex with the given parabola.
I mentioned earlier that I would try to come up with an easier way to determine the final graph. After much searching, I can't seem to discover this. Instead, I have decided to try one more thing. I want to try to turn the parabolas 90 degrees.


I switched the x and y variables and the graphs were turned, but they were rotated 90 degrees around the origin and I wanted to rotate them 90 degrees around the shared vertex. My next attempt will be to get all 4 parabolas to share the same vertex.


This is the graph that I have to start out with. All four parabolas are present and the only difference is that the y's and x's have been interchanged. Now I must try to move the two new parabolas to share a vertex with the original two. I will begin with moving one at a time and will simply allow the other to disappear for a while.


I began changing the (+6) to smaller integers and the graph did begin to move to the left. I realized that I would need a negative number, so I began trying some. I stopped with (-3) for now. My plan is to try to move the graph up towards the original two.


It's very hard for me to put into words, but I discovered that whatever moved the original 2 parabolas, did the "opposite" to the new ones. So in order to move the parabola up, I needed to change the "y" variable to (y-5 1/2). Now I leave this parabola alone for a while and try to work with the other parabola.


I just wanted to show a middle step. I changed the (+) to a (-6) to move the parabola to the left.


This was the "easy" move, because I knew that the two parabolas had to be at the same point on the y axis, so I changed the "y" to (y-5 1/2) in this formula too. Now my objective it to simply use what I know about how the parabolas move to try to manuver the 2 new ones to share the same vertex with the 2 original ones.



If you notice, I had to also change the 5 1/2 to 5 5/8 to move the parabolas up just a little. I am so happy with myself. I figured out how to do what I set out to do.
This was a very interesting problem to try.