

Assignment 9
1a. Let triangle ABC be any triangle. Then if P is any point in the plane, then the triangle formed by constructing perpendiculars to the sides of ABC (extended if necessary) locate three points R, S, and T that are the intersections. Triangle RST is the Pedal Triangle for Pedal Point P.
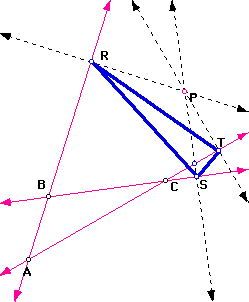
1b. Use GSP to create a script for the general construction of a pedal triangle to triangle ABC where P is any point in the plane of ABC.
Click here to see the SCRIPT for a Pedal Triangle. Go ahead, explore it for yourself...make sure to choose four points before you begin! What do you see?
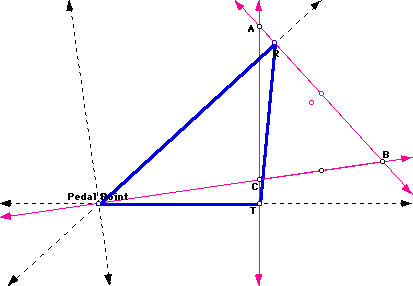
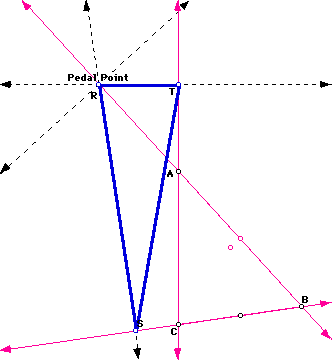
The pictures above are quite nice, but why don't you click here to look at the animation of all the movements of the Pedal Triangle. Do you see anything different?
2. What if pedal point P is the centroid of triangle ABC?
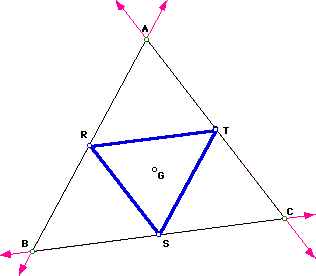
If and when pedal point P is the centroid of triangle ABC, then the pedal triangle becomes the medial triangle.
3. What if . . . P is the incenter . . . ?
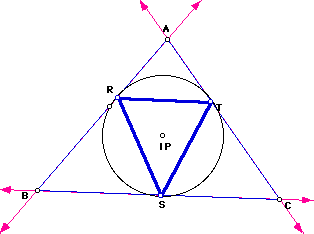
If pedal point P is the incenter of triangle ABC, then all three vertices of the pedal triangle lie on the segments of triangle ABC and they are the points of intersection of the incircle.
4. What if . . . P is the Orthocenter . . . ? Even if outside ABC?
Inside:
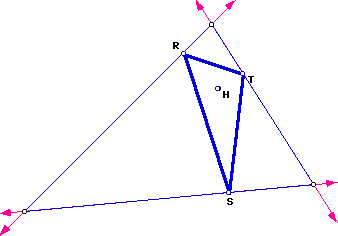
When pedal point P is the orthocenter of triangle ABC, then the pedal triangle becomes the orthic triangle.
Outside:
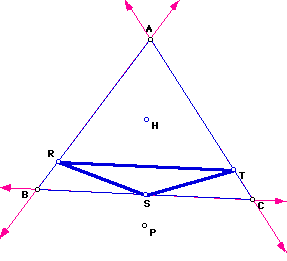
Look. Pedal point P must remain inside triangle ABC!
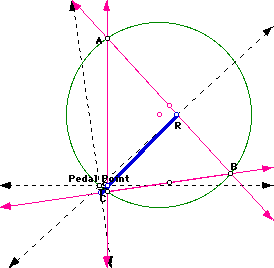
5. What if . . . P is the Circumcenter . . . ? Even if outside ABC?
Inside:
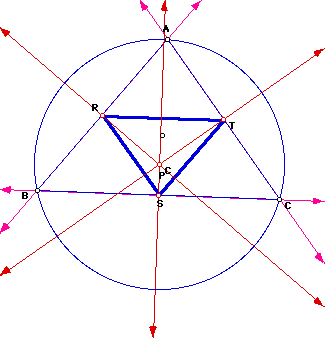
Notice that pedal point P and the circumcenter lie on the same location here. What do you see here, other than the obvious information that I have given? Do you see that all three points of the pedal triangle lie on the circumcircle? Did you also notice that the perpendicular bisectors of each side of triangle ABC also bisect points R, S, and T? You can see this more thoroughly if you click here and then double click the animate circumcircle.
Outside:
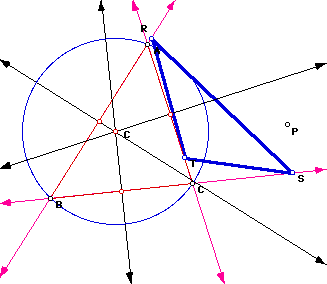
Whether pedal point P lies outside the circumcircle or triangle ABC, P will no longer lie along the same path as the circumcenter.
6. What if . . . P is the Center of the nine point circle for triangle ABC?

When pedal point P lies on the center of the nine point circle, then all points of the pedal triangle also seem to lie on this circle. The pedal triangle also seems to become an isosceles triangle.
7. What if P is on a side of the triangle?
What do you think may happen when and if pedal point P is on any side of triangle ABC? Let's find out from this construction:
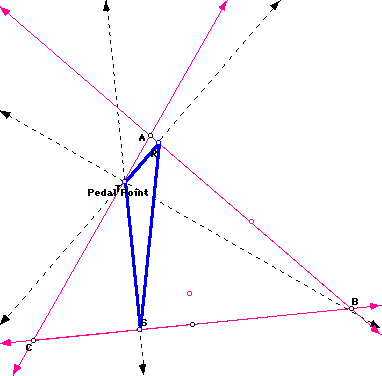
If pedal point P is on a side of triangle ABC, then point P's perpendicular line (the dashed line) is also moving along that line. Also, look at where Pedal Point P is in the picture above. It lies directly on top of one of the pedal triangle's points. (This will occur for all sides).
8. What if P is one of the vertices of triangle ABC?
Let's take a look and see!
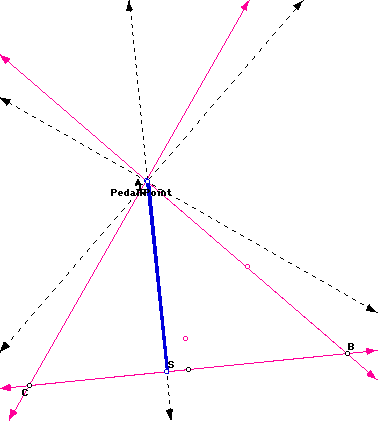
As pedal point P lies on one of the vertices of any triangle ABC, the Simson Line is created. Look at the pedal triangle!!! It is a straight line!!! It is also the altitude of this triangle.
9. Find all conditions in which the three vertices of the Pedal triangle are colinear (that is, it is a degenerate triangle). This line segment is called the Simson Line.
When all three vertices of the Pedal triangle are colinear and are said to be a "degenerate triangle" or a line segment or the given name of Simson Line, the pedal point P will lie on the circumcircle. To see this construction again, click here.