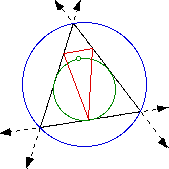

In the preceding diagram, the green circle is the nine-point circle of
the black triangle, whose sides have been extended as dashed lines. The
red triangle is the Pedal Triangle of the point on the green circle. The
blue circle is the circumcircle of the black triangle. The nine-point circle
was chosen for the location of the Pedal Point P, because it can intersect
the circumcircle of the given triangle. The nine-point circle can intersect
the circumcircle in two places or it can be tangeant to the circumcircle
at one point. Below are two ways that a nine-point circle can intersect
the circumcircle.
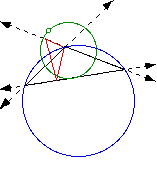
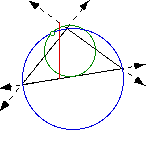

Notice that if P is located upon the circumcircle, the resulting Pedal
Triangle is the Simpson Line (all the vertices are colinear). If the sides
of the Pedal Triangle are extended as lines, it is very easy to see that
they are colinear.
These lines can be traced, as P is moved along the nine-point circle,
with the following result. The narrowest point between the two sides of
the curve seem to be defined when the Pedal Triangle has degenrated into
the Simpson Line.


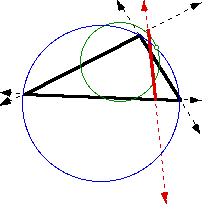
By examining the case when the nine-point circle and the Circumcircle
are tangent (above), it can be seen that the width narrowest point is not
bordered by Simpson Lines on both sides, because in this case there is only
one Simpson Line, and the narrowest point of the path of the Simpson Lines
is wider.