

It has now become a rather standard exercise, with the available technology,
to construct graphs to consider the equation
and to overlay several graphs of
for different values of a, b, or c as the other two are held constant.
From these graphs discussion of the patterns
for the roots of
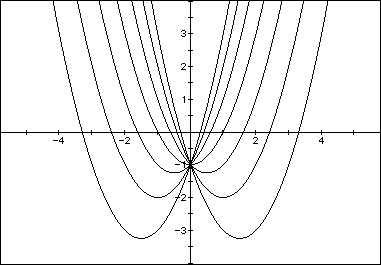
can be followed. For example, if we set
for b = -3, -2, -1, 0, 1, 2, 3, and overlay the graphs, the following picture
is obtained.

We can now discuss the "movement" of a parabola as b is
varied while keeping both a and c constant.
1. All these parabola's have one point in common, (0,1) (the point where each parabola crosses the y-axis).
2. For b<-2, the parabola intersects the x-axis in two points with positive x values (i.e. the original equation will have two real roots, both positive).
3. For b=-2, the parabola is tangent to the x-axis and so the original equation has one real(positive) root at the point of tangency.
4. For -2<b<2, the parabola does not intersect the x-axis -- the original equation has no real roots.
5. For b=2, the parabola is tangent to the x-axis and so the original equation has one real(negative) root at the point of tangency.
6. For b>2, the parabola intersects the x-axis in two points with negative x values (i.e. the original equation will have two real roots, both negative).
Consider a version of the equation above
We will now graph this equation in the xb-plane. We get the following graph.

If we take any particular value of b, say b = 3, and overlay
this equation on the graph above we add a line parallel to the
x-axis. If this line intersects the curve in the xb plane, the intersection
points correspond to the root(s) of the original
equation for that value of b. The graph with b = 3 overlayed looks
like the following.
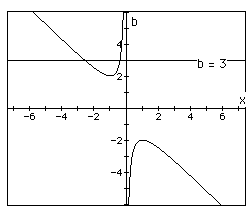
For each value of b we select, we get a horizontal line. It is clear from this graph that we get two negative real roots of the original equation when b > 2, one negative real root when b = 2, no real roots for -2 < b < 2, one positive real root when b=-2, and two positive real roots when b < -2.
Viewing the quadratic in the xb-plane allows one to see more clearly that there are no real roots on the interval -2<b<2. The graph below illustrates this point by overlaying the equations b=1 and b=-1 onto the xb-plane which already shows the graph of the quadratic.
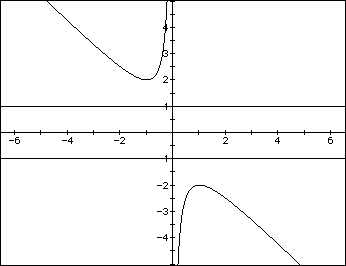
From this graph one can see that since the graphs of b=-1 and b=1 do not cross the graph of the quadratic that there are no real roots associated with the quadratic with b=-1 and b=1.
Now consider the case when c = - 1 rather than c = 1.
Overlaying graphs of this equation in the xy-plane for b={-3, -2, -1, 0, 1, 2, 3} gives you the following.
Now graphing the quadratic in the xb-plane yields
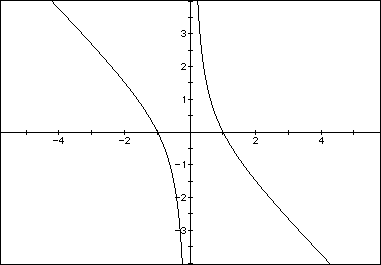
In contrast to the graph in the xy-plane, this graph makes it easy to see that for any value of b you get two real roots, one positive and one negative.
We will now move on to the case when c is varied and a and b are held constant. We will use the following equation in this example:
If the equation is graphed in the xc-plane,
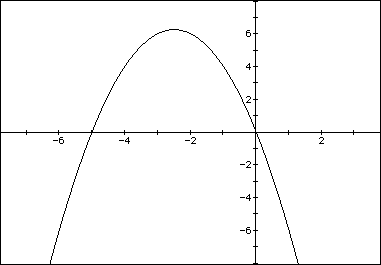
it is easy to see that the curve will be a parabola. For each value of c considered, its graph will be a line crossing the parabola in 0, 1, or 2 points -- the intersections being at the roots of the orignal equation at that value of c. In the graph below, the graph of c = 1 is overlayed on the previous graph.
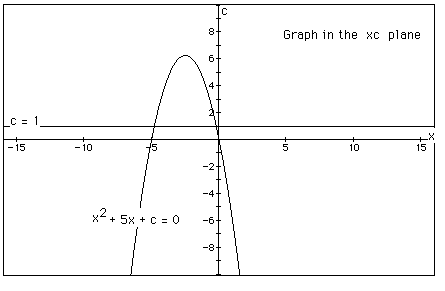
From the two previous graphs one can see, there is one value of c where the equation will have only 1 real root -- at c=6.25. Also, for c > 6.25 the equation will have no real roots and for c < 6.25 the equation will have two roots. Both roots will be negative for 0 < c < 6.25, one root will be negative and one 0 when c = 0 and one root will be negative and one positive when c < 0.
This equation,![]() , will have two negative roots -- approximately -0.2
and -4.8.
, will have two negative roots -- approximately -0.2
and -4.8.
The graphs in the xa-plane are nearly as easy to read as those in the xb and xc. We have not explored the xa-plane here; however, we leave it to the reader to do this exploration if his/her curiousity is running wild.
We have just demostrated some different ways of looking at the quadratic
for different values of a, b and c and determining what type(s) of roots the equation will yield. Viewing the quadratic in the xb or xc-plane, may make determining roots easier for some people. If you are comfortable with determining roots using the xy-plane that is great but you should still understand these other methods and vice-versa. It is worth understanding how many different methods work even if you prefer one over the other(s).