

The spreadsheet is a utility tool that can be adapted to many different explorations, presentations, and simulations in mathematics. An essential feature should be the ability to make graphs and charts from the matrix of data. Generate the Fibonnaci sequence in the first column using f(0) = 1, f(1) = 1, f(n) = f(n-1) + f(n-2).
The Fibonacci sequence of numbers has been studied for hundreds of years for his mathematical beauty and its appearance in nature and the man-made world. This investigation will begin with the basics of generating the Fibonacci sequence and continue to explore the golden ration, the Lucas sequence and the existence of the sequence in the world around us.
Let's begin with a bit of history of the number sequence.The sequence was named for the mathematician known as Leonardo of Pisa or Fibonacci. He lived in the 1400's in Italy, but traveled many places learning about math, wherever he went. He is credited with 'discovering' the number sequence named for him, but it is difficult to determine if he was the first to ever study it.
The formula to generate the Fibonacci sequence is as follows:
Generating this sequence by hand is beneficial, yet a spreadsheet can help to continue the sequence for larger terms and also help to manipulate in order to recognize any relationships.
The following is the first 50 terms of the Fibonacci sequence.
| Term | |
| 1 | 1 |
| 2 | 1 |
| 3 | 2 |
| 4 | 3 |
| 5 | 5 |
| 6 | 8 |
| 7 | 13 |
| 8 | 21 |
| 9 | 34 |
| 10 | 55 |
| 11 | 89 |
| 12 | 144 |
| 13 | 233 |
| 14 | 377 |
| 15 | 610 |
| 16 | 987 |
| 17 | 1597 |
| 18 | 2584 |
| 19 | 4181 |
| 20 | 6765 |
| 21 | 10946 |
| 22 | 17711 |
| 23 | 28657 |
| 24 | 46368 |
| 25 | 75025 |
| 26 | 121393 |
| 27 | 196418 |
| 28 | 317811 |
| 29 | 514229 |
| 30 | 832040 |
| 31 | 1346269 |
| 32 | 2178309 |
| 33 | 3524578 |
| 34 | 5702887 |
| 35 | 9227465 |
| 36 | 14930352 |
| 37 | 24157817 |
| 38 | 39088169 |
| 39 | 63245986 |
| 40 | 102334155 |
| 41 | 165580141 |
| 42 | 267914296 |
| 43 | 433494437 |
| 44 | 701408733 |
| 45 | 1134903170 |
| 46 | 1836311903 |
| 47 | 2971215073 |
| 48 | 4807526976 |
| 49 | 7778742049 |
| 50 | 12586269025 |
It is interesting to note that Fibonacci numbers, such as 2,3,5,8,13, 21,...have been found in many facets of nature. Some examples include the petals and stems of flowers; the number of seeds in a sunflower; the leaves in pine cones, pineapples and artichokes; and the number of sections in a grapefruit.
The Fibonacci sequence also generates the golden ratio. This ratio can be created by dividing successive numbers of the sequence. The ratio appears as n increases.
The golden ratio is equal to approximately 1.618....It
is a repeating decimal with no known pattern. Another way to write the golden
ratio is ![]() .
.
The spreadsheet is able to calculate the ratio for any number of terms.
| 1 | |
| 2 | 1 |
| 3 | 2 |
| 4 | 1.5 |
| 5 | 1.66666666666667 |
| 6 | 1.6 |
| 7 | 1.625 |
| 8 | 1.61538461538462 |
| 9 | 1.61904761904762 |
| 10 | 1.61764705882353 |
| 11 | 1.61818181818182 |
| 12 | 1.61797752808989 |
| 13 | 1.61805555555556 |
| 14 | 1.61802575107296 |
| 15 | 1.61803713527851 |
| 16 | 1.61803278688525 |
| 17 | 1.61803444782168 |
| 18 | 1.61803381340013 |
| 19 | 1.61803405572755 |
| 20 | 1.61803396316671 |
| 21 | 1.6180339985218 |
| 22 | 1.61803398501736 |
| 23 | 1.6180339901756 |
| 24 | 1.61803398820532 |
| 25 | 1.6180339889579 |
| 26 | 1.61803398867044 |
| 27 | 1.61803398878024 |
| 28 | 1.6180339887383 |
| 29 | 1.61803398875432 |
| 30 | 1.6180339887482 |
It might be helpful to see how each successive numbers tend toward the golden ratio as n increase with a graph.
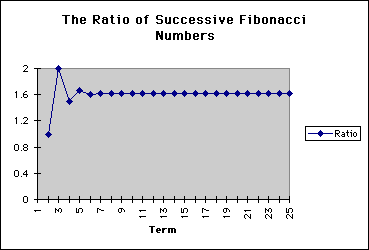
There are other sequences of numbers that follow the generating method of the Fibonacci sequence. The sequence begins with f(0) = 2. The following table has only the first 15 terms (to save space).
| 1 | 2 |
| 2 | 2 |
| 3 | 4 |
| 4 | 6 |
| 5 | 10 |
| 6 | 16 |
| 7 | 26 |
| 8 | 42 |
| 9 | 68 |
| 10 | 110 |
| 11 | 178 |
| 12 | 288 |
| 13 | 466 |
| 14 | 754 |
| 15 | 1220 |
The ratio, however, between successive numbers is the same. As n increases the ratio tends towards the golden ratio. In fact, the list of ratios is an exact replica of the ratios of the Fibonacci sequence.
| 2 | |
| 2 | 1 |
| 4 | 2 |
| 6 | 1.5 |
| 10 | 1.66666666666667 |
| 16 | 1.6 |
| 26 | 1.625 |
| 42 | 1.61538461538462 |
| 68 | 1.61904761904762 |
| 110 | 1.61764705882353 |
| 178 | 1.61818181818182 |
| 288 | 1.61797752808989 |
| 466 | 1.61805555555556 |
| 754 | 1.61802575107296 |
| 1220 | 1.61803713527851 |
| 1974 | 1.61803278688525 |
| 3194 | 1.61803444782168 |
| 5168 | 1.61803381340013 |
| 8362 | 1.61803405572755 |
| 13530 | 1.61803396316671 |
| 21892 | 1.6180339985218 |
| 35422 | 1.61803398501736 |
| 57314 | 1.6180339901756 |
Looking at various number sequences such as adding each successive odd numbers (starting with the first two numbers) together produces the same result when determining their ratios.
| 1 | 1 | |
| 2 | 3 | 3 |
| 3 | 4 | 1.33333333333333 |
| 4 | 7 | 1.75 |
| 5 | 11 | 1.57142857142857 |
| 6 | 18 | 1.63636363636364 |
| 7 | 29 | 1.61111111111111 |
| 8 | 47 | 1.62068965517241 |
| 9 | 76 | 1.61702127659574 |
| 10 | 123 | 1.61842105263158 |
| 11 | 199 | 1.61788617886179 |
| 12 | 322 | 1.61809045226131 |
| 13 | 521 | 1.61801242236025 |
| 14 | 843 | 1.61804222648752 |
| 15 | 1364 | 1.61803084223013 |
| 16 | 2207 | 1.61803519061584 |
| 17 | 3571 | 1.6180335296783 |
| 18 | 5778 | 1.61803416409969 |
| 19 | 9349 | 1.61803392177224 |
| 20 | 15127 | 1.61803401433308 |
| 21 | 24476 | 1.61803397897799 |
| 22 | 39603 | 1.61803399248243 |
| 23 | 64079 | 1.61803398732419 |
| 24 | 103682 | 1.61803398929446 |
Adding the even numbers (starting with the first two numbers) together produces this list of numbers
| 1 | 2 | |
| 2 | 4 | 2 |
| 3 | 6 | 1.5 |
| 4 | 10 | 1.66666666666667 |
| 5 | 16 | 1.6 |
| 6 | 26 | 1.625 |
| 7 | 42 | 1.61538461538462 |
| 8 | 68 | 1.61904761904762 |
| 9 | 110 | 1.61764705882353 |
| 10 | 178 | 1.61818181818182 |
| 11 | 288 | 1.61797752808989 |
| 12 | 466 | 1.61805555555556 |
| 13 | 754 | 1.61802575107296 |
| 14 | 1220 | 1.61803713527851 |
| 15 | 1974 | 1.61803278688525 |
| 16 | 3194 | 1.61803444782168 |
| 17 | 5168 | 1.61803381340013 |
| 18 | 8362 | 1.61803405572755 |
| 19 | 13530 | 1.61803396316671 |
| 20 | 21892 | 1.6180339985218 |
| 21 | 35422 | 1.61803398501736 |
| 22 | 57314 | 1.6180339901756 |
| 23 | 92736 | 1.61803398820532 |
| 24 | 150050 | 1.6180339889579 |
| 25 | 242786 | 1.61803398867044 |
Another ratio to investigate is the ratio of every second term. For space, I have placed only the table of the Fibonacci numbers below. Observe any similarities to the ratio of each successive numbers.
| 1 | |
| 1 | |
| 2 | 2 |
| 3 | 3 |
| 5 | 2.5 |
| 8 | 2.66666666666667 |
| 13 | 2.6 |
| 21 | 2.625 |
| 34 | 2.61538461538462 |
| 55 | 2.61904761904762 |
| 89 | 2.61764705882353 |
| 144 | 2.61818181818182 |
| 233 | 2.61797752808989 |
| 377 | 2.61805555555556 |
| 610 | 2.61802575107296 |
| 987 | 2.61803713527851 |
| 1597 | 2.61803278688525 |
| 2584 | 2.61803444782168 |
| 4181 | 2.61803381340013 |
The ratio of each successive term tends toward the number 2.618...Notice that it is similar to the golden ratio. The relationship between these two numbers (1.618) and (2.1618) is extraodinary. It is easy to see that they have an arithmetic difference of 1, but 2.618 is also the square of 1.618. Thus, you can say that the golden ratio's square is equal to the golden ratio plus 1.
| 1 | ||||
| 1 | ||||
| 2 | 2 | 3 | 5 | 8 |
| 3 | 3 | 5 | 8 | 13 |
| 5 | 2.5 | 4 | 6.5 | 10.5 |
| 8 | 2.66666666666667 | 4.33333333333333 | 7 | 11.3333333333333 |
| 13 | 2.6 | 4.2 | 6.8 | 11 |
| 21 | 2.625 | 4.25 | 6.875 | 11.125 |
| 34 | 2.61538461538462 | 4.23076923076923 | 6.84615384615385 | 11.0769230769231 |
| 55 | 2.61904761904762 | 4.23809523809524 | 6.85714285714286 | 11.0952380952381 |
| 89 | 2.61764705882353 | 4.23529411764706 | 6.85294117647059 | 11.0882352941176 |
| 144 | 2.61818181818182 | 4.23636363636364 | 6.85454545454545 | 11.0909090909091 |
Notice the last line of the table. This is the value that each ratio tends towards as n increases. It also has the distinction of being the golden ratio squared, cubed, quadrupled and quintupled.
The use of spreadsheets can peak interest into the patterns and mathematical basis for the golden ratio. The discussion that follows is a nice connection back to the Fibonacci sequence.
As mentioned above, the golden ratio has the distinction of having its square equal to itself plus one. The support for this fact can be found when we look at a line segment that has been parted into the golden ratio.
Any line segment that has been divided into the golden segment can be written as the proportion
If we substitute
We find
Multiplying each side by x, gives us
To connect this finding to the Fibonacci sequence continue to find the "values" of the powers of x (assuming that x is representative of the golden ratio).
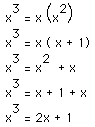 |
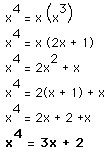 |
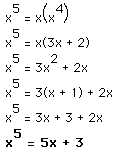 |
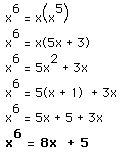 |
Notice that the coefficients in each term are successive Fibonacci numbers. This patterns continues and is consistent for each power of x.