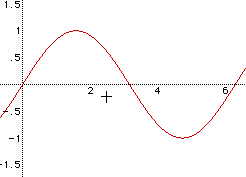
It is typical in a Trigonometry class to discuss the sine function and
the various attributes of its graph. The graph of y = sin x is referred
to as the "parent" graph and will be the graph to which all of
our comparisons are made. One period of the function y = sin x looks like
this:

Let's see what would happen if a negative were located in front of this
function.
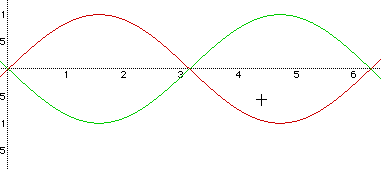
In the above picture, the red graph represents the function y = sin x
and the green graph represents the function y = - sin x. Although the x-intercepts
did not change, it appears as though each y-ccordinate is being multiplied
by a negative 1. Hence, a negative value reflects the graph about the x-axis.

Notice that the graph is red is y = sin x and this represents our "parent"
graph, whereas the green graph is y = 2 sin x. Although the x-intercepts
do not change, it appears as though each y-intercept from the parent graph
is being multiplied by 2. It follows that if the amplitude (or height) of
y = sin x is 1 then y = 2 sin x has an amplitude of 2. Hence, varying the
the value for a affects the height of the graph. At this point, let's vary
the value of b when y = a sin(bx + c).
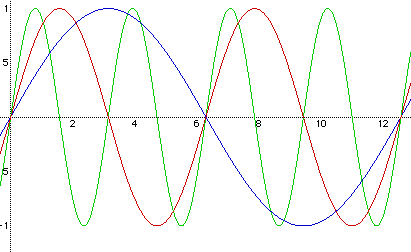
The red graph represents the function y = sin x which is our "parent" graph; the blue graph represents the function
One obvious difference is that the period (or one complete cycle) of y = sin x is given by
or approximately 6.28. Notice that the blue graph has a period of ![]() ,
where we are simply taking the standard period and multiplying
,
where we are simply taking the standard period and multiplying ![]() times 2. It follows that the graph is stretched and the coefficient of x
(which is (1/2))is causing this stretch. Furthermore, the green graph represents
the function y = sin 2x. Notice here that the period or cycle has been reduced.
One may easily look at the graph and notice that the period has been reduced
from
times 2. It follows that the graph is stretched and the coefficient of x
(which is (1/2))is causing this stretch. Furthermore, the green graph represents
the function y = sin 2x. Notice here that the period or cycle has been reduced.
One may easily look at the graph and notice that the period has been reduced
from ![]() to just
to just ![]() . Hence, the coefficient of x affects the stretching
or shrinking of the graph. Indeed, if x has an integral coefficient then
the graph will undergo a horizontal shrink, whereas if x has a fractional
coefficient then the graph will undergo a horizontal stretch. In addition,
the "critical" values along the x-axis are altered as well. The
"parent" graph y = sin x has critical values of 0, (pi)/2, pi,
(3 pi)/2, and 2 pi. As for the function y = sin (1/2)x, the new critical
values are 0, pi, 2 pi, 3 pi, 4pi. It would appear as though each critical
value along the x-axis is being multiplied by a factor of 2. If we examine
the critical values of y = sin 2x, we notice that each critical value along
of the parent graph is being multiplied by a factor of (1/2), which is equivalent
to dividing each value by 2. Those critical values are 0, (pi)/4), (pi)/2,
(3 pi)/4, and pi. Notice that the coefficient of x directly affected the
period, but had no bearing on the amplitude whatsoever.
. Hence, the coefficient of x affects the stretching
or shrinking of the graph. Indeed, if x has an integral coefficient then
the graph will undergo a horizontal shrink, whereas if x has a fractional
coefficient then the graph will undergo a horizontal stretch. In addition,
the "critical" values along the x-axis are altered as well. The
"parent" graph y = sin x has critical values of 0, (pi)/2, pi,
(3 pi)/2, and 2 pi. As for the function y = sin (1/2)x, the new critical
values are 0, pi, 2 pi, 3 pi, 4pi. It would appear as though each critical
value along the x-axis is being multiplied by a factor of 2. If we examine
the critical values of y = sin 2x, we notice that each critical value along
of the parent graph is being multiplied by a factor of (1/2), which is equivalent
to dividing each value by 2. Those critical values are 0, (pi)/4), (pi)/2,
(3 pi)/4, and pi. Notice that the coefficient of x directly affected the
period, but had no bearing on the amplitude whatsoever.
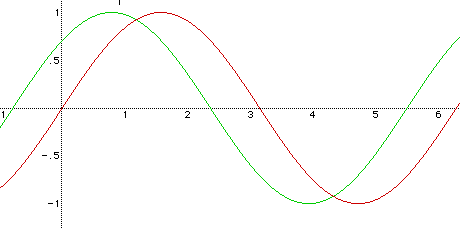
What changed here? Indeed, we are able to look at the graph and notice
that the amplitude remained unchanged and the period is still 2 pi. However,
we can readily see that the graph of the function y = sin (x + (pi)/4) (in
green) and its critical values have been shifted to the left simply by comparing
the green graph with the parent graph. Indeed, the graph shifted (pi/4)
units to left just by adding (pi/4). It seems somewhat counter-intuivtive
in that a student would typically think that adding a value would transform
the graph in a positive direction. Let's see what happens if we subtract
some value. Will the transformation be to the right in the positive direction?
Again, let's compare the parent graph with y = sin (x - pi). If our hypothesis
is correct, the graph should be transformed pi units to the right. Notice
the graph below.
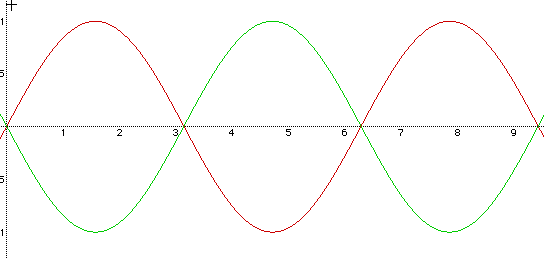
Fortunately, the conjecture was correct. Notice that our parent graph
in red has an initial position of 0 and a terminal position of 2 pi if we
only want to examine one period of the function; however, the green graph
has has an initial position of pi and a terminal position of 3 pi. Note
that all of the critical values from the parent to y = sin (x + pi) may
be found by adding pi to each value--hence transforming the graph pi units
to the right.
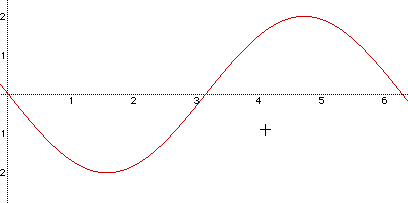
*If we read the rule from left to right, we first notice that there is
a negative in front of the function so there should be a reflection over
the x-axis. Furthermore, the 2 in front of the function should alter the
amplitude by multiplying each y-coordinate by 2. The period of 2 pi will
not change and there will be no phase shift. Does the graph below fit this
description?
*In this case, the (1/3) will affect the amplitude by dividing each y-coordinate
by 3. So, instead of the range being [-1,1] as with the parent graph, the
range should by [(-1/3), (1/3)]. Also, the normal period of 2 pi will be
affected as well due to the fact that x has a coefficient other than 1.
We noted earlier that if the coefficient were an integer, then the graph
would undergo a horizontal shrink and in this case our new period would
be pi as opposed to 2 pi. Finally, this graph will be shifted pi/4 units
to the right. Indeed, it would not be terribly difficult to very this description
by using Algebra Xpresser.
 \
\Obviously, this holds true.
The attributes of a sine function may be described in the following manner.
If
y = a sin (bx + c), a is called the amplitude and is given by |a|. The period
of the sine function is normally 2pi; however, the period of the function
may be found by ((2pi)/b). The phase shift may be found by setting-up the
following equation: bx + c = 0. In fact, solving this equation for x will
yield the initial position for the function and solving the equation bx
+ c = 2pi will provide the ending position (for one period of the graph).