Click here for a problem description
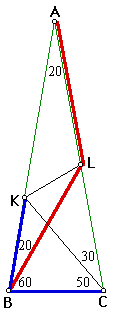
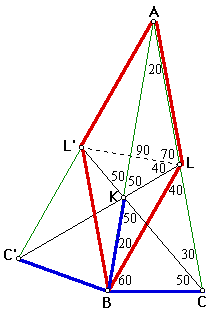
This problem, like the 100 degree isosceles triangle problem can frustrate one for a long time and when you finally find a solution you may be disappointed by its "simplicity."
A solution......
Reflect the entire sketch about line AB, this creates the kite ALBL' with AB and LL' intersecting at 90 degrees.
It remains to fill in the missing angles and determine the size of the angle of interest:
angle KLB = 30 degrees


As with so many of these problems a clever construction and the problem is not as bad as it seems at first.
Construct AD so that angle BAD = 60 degrees and AD = AM.
It follows that angle DAC = 80 degrees, and by the SAS case of congruency; triangle CAD is congruent to triangle ABC, from which it follows that DC = AC (since AC = AB - given).
Now by the SSS case of congruency triangle AMC is congruent to triangle DMC, and it follows that:
angle ACM = 10 degrees