Determine the ratio of the interior "rounded square" to the area of the square.
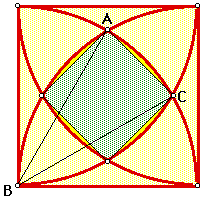Consider the problem as follows:
We can determine AC using the cosine rule, with AB = AC = S (the side of the square), and angle ABC = 30 deg.
and the area of the interior square follows:
The area of one yellow arc can be thought of as the area of sector BAC minus the area of triangle ABC:
And the ratio of the area of the interior region to the area of the square follows:
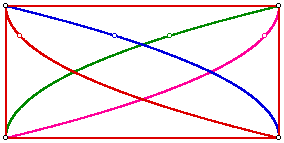This problem is very similar - we use a rectangle and instead of using
circles to make the acrs we use parabola as illustrated.
The problem is still to find the ratio of the interior are to the are of the rectangle.
Click on the GSP icon to access a script
that will draw the sketch given two vertices of the rectangle.
