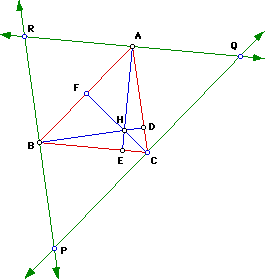

Given triangle ABC with altitudes: AE, BD and CF we want to show that
they meet in one point.
Through each of the vertices of the triangle construct a line parallel to
the opposite side of the triangle forming triangle PQR.
Now RA = BC since RACB is a parallelogram,
Also AQ = BC since ABCQ is a parallelogram,
Hence RA = AQ
AE is perpendicular to RQ since AE is perpendicular to BC and BC is parallel
to RQ
Hence AE is the perpendicular bisector of RQ.
Similarly BD is the perpendicular bisector of RP and CF the perpendicular
bisector of QP.
AE, BD and CF are therefore concurrent since we know that the perpendicular
bisectors of the sides of a triangle are concurrent at a point called the
circumcenter (C)