
10-Day Unit:
Geometric Exploration of Circles
by
Jennifer Roth
I am assuming that the students have worked with GSP before, and they
have already done investigations with triangles and polygons. I am also
assuming that each student has access to a computer containing the GSP software.
Unit Objective: Understand several of the geometric properties of
circles.
Lesson One:
(This lesson will probably last 1 class period)
Goal: The students will be able to inscribe and circumscribe circles about
several different polygons.
This lesson is used as an introduction to circle that makes connections
to their previous knowledge of triangles and polygons.
Class Exercise:
I will give the students the following exercises to complete using GSP
1. Construct a scalene triangle. Construct its inscribed circle
2. Construct a scalene triangle. Construct its circumscribed circle.
3. Construct a square. Construct its inscribed circle.
4. Construct a square. Construct its circumscribed circle.
5. Construct a rectangle. Can you constuct its inscribed circle or circumscribed
circle?
6. Construct a nonisosceles trapezoid. Can you constuct its inscribed circle
or circumscribed circle?
7. Construct an isosceles trapezoid. Can you constuct its inscribed circle
or circumscribed circle?
8. Pick any other polygon. Can you constuct its inscribed circle or circumscribed
circle?
The students will be able to work together, and when they are finished I
will split them up into groups. And I will as them to write what conjectures
they can make about inscribing and circumscribing circles and quadrilaterals.
And I will ask them to write what conclusions they can make about the polygons
they chose.
Lesson Two:
(This lesson will probably last 1 class period)
Goal: The students will be able to use the vocabulary used to describe
the circle.
I will introduce the following terms with the following pictures up on the
display screen.
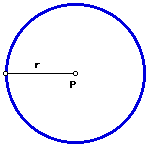
Circle and Radius

Diameter

Chord
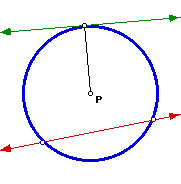
Tangent, Point of Tangency, Secant
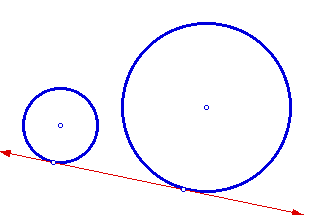
Common external tangent
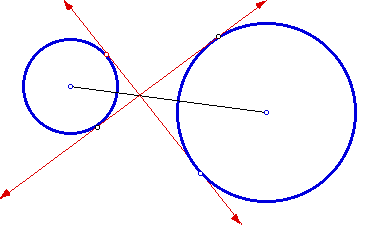
Common internal tangents
Class Exercise:
I will have the following circle set up on their computer for them to find
the radius and diameter.
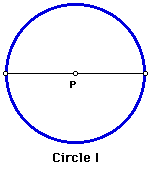
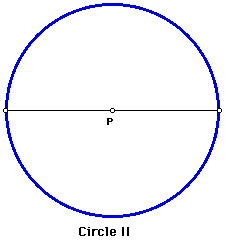
I will split the class up into groups and ask them to make conjecture about
the relationship between the radius and diameter of a circle. I will also
ask them to remain in their groups and come up with the different ways that
2 circlet intersect.
We will discuss as a group, and I will define the terms Tangent Circles,
Concentric Circles, and Congruent Circles.
Lesson Three:
(This lesson will probably last 2 class periods)
Goal: The students will be able to use properties of tangents to solve problems.
Class Exercise:
I will have the following construction on all the student computers.
Construct a circle with center P. Construct a tangent line to the circle.
Label the point of tangency Q.

I will ask the students to measure the angles made by the radius. Then,
I will ask them to write down what conclusions they cam make about the tangent
lin in relation to the radius.
We will get together and discuss as a class.
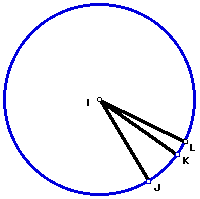
I will also have the following construction on their computer.

I will ask them to use this diagram to find the following segment lenghts.
1. KN
2. JP
3. LO
4. KL
5. KO
6. LN
7. PO
8. KM
They will turn their answers in to me.
I will ask the students to complete the following construction.
Construct a circle with center P. Construct points R and T on the circumference
of the circle. Construct the tangent lines to these points, and label their
intersection point S.

I will ask the students to make conclusions about segments ST and SR.
They will be allowed to work together, and then we will discuss solutions
as a class.
Then, I will ask them to prove the Theorem: If two segments from the same
exterior point are tangent to a circle, then they are congruent. And, I
will ask them to turn their solutions in to me.
Homework: I will give them a proof involving tangents, and I will
give them problems in which they are to find a segment length using the
Pythagorean Theorem and their knowledge of tangents.
Lesson Four:
(This lesson will probably last 1 day)
Goal: The students will be able to measure central angles and arcs of circles.
I will introduce the following terms with the following pictures up on the
display screen.
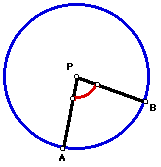
Central Angle
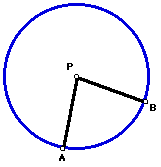
Minor Arc
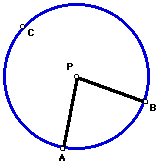
Major Arc
I will explain the Solution to the following example
EXAMPLE: A Circle is circumsribes about an equilateral triangle, RST. Find
the measures of the minor arc RS and the major arc RTS

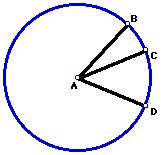
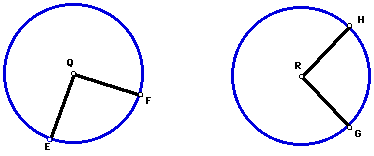
I will have the following circles on the student computers and I will
ask them to measure the adjacent arcs. Then, I will split them up into groups
and ask them to make conclusions.

We will then discuss the conclusions as a class.
I will have the following on each of the student computers


I will ask the students to measure arcs and angles, tanslate points and
make more measurements, and make conclusion. Click
here to view the GSP file that I will have on the student computers.
We will discuss the conclusions as a class.
Homework: I will give the students problems involving recognition of
minor and major arcs, and finding arc and angle measurements on a given
circle. I will give them a problem involving a clock and finding measurements
on it.
Lesson Five:
(This lesson will probably last 2 days)
Goal: The students will be able to use properties of chords and arcs to
solve problems.
I will have the following constructions on the student computers
Construction 1:
Construct a circle with center P. Construct 2 congruent chords AB and CD.
Construct radii AP, BP, CP, and DP.

I will ask them to measure angle APB and angle CPD, and make conclusions.
Construction 2:
Construct a circle with center Q. Consruct a chord that is not the diameter
and label it AB. Construct a line perpendicular to AB through P. Label the
point of intersection C.

I will ask the students to compare lenght AC with CB and make conclusions.
We will discuss the results as a class.
I will go over the following example with the class
EXAMPLE:

In the above figure, RS and TU are chords of the larger circle and tangents
of the smaller circle. The two circles are concentric. I will explain why
RS and TU are congruent.
I will give the class problems involving chords and arcs to work on together
in groups, and then I will have the groups present their problems to the
class.
Lesson Six:
(This lesson will probably last 1 day)
Goal: The students will be able to use inscribed angles to solve problems.
I will define Inscribed angle and Intercepted arc for the students.
I will have them complete the following construction and make conclusions.
Construct a circle with center P. Construct a central angle RPS. Construct
three other points, T, U, V, on the circumference of the circle. Construct
angles RTS, RUS and RVS. Measure the 4 angles and make conclusion.
We will discuss th conclusions as a class.
I will go through the following example:
EXAMPLE:
Find the measure of the angles that form the points of a regular 5-pointed
star.
I will ask the class to prove the following theorems:
1. If two inscribed angles of a circle intercept the same arc, the angles
are congruent
2. An angle that is inscribed in a circle is a right angle if and only if
its corresponding arc is a semicircle.
3. A quadrilateral can be inscribed in a circle if and only if its opposite
angles are supplementary.
Homework: I will give the students problems involving finding the measure
of angles and arcs, and involving if a circle can be circumscribed about
a given quadrilateral.
Lesson 7:
(This lesson will probably last 1 day)
Goal: The students will be able to measure angles formed by tangents, chords,
and secants.
I will introduce the following theorems.
1. If a tangent and a chord intersect at a point on a circle, then the measure
of each angle formed is half the measure of the intercepted arc.
2. If two chords intersect in the interior of a circle, then the measure
of each angle is half the sum of the measure of the arcs intercepted by
the angle and its vertical angle.
3. If a tangent and a secant, two tangents, or two secants intersect in
the exterior of a circle, then the measure of the angle formed is half the
difference of the measure of the intercepted arcs.
I will give students the following problems to work on and present to class.
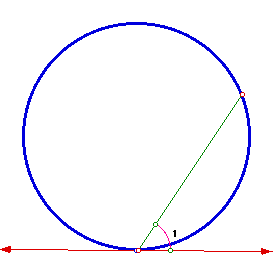
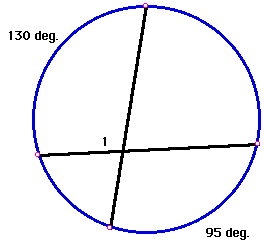
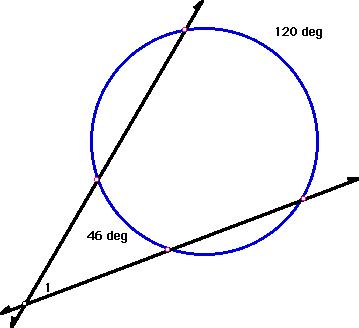
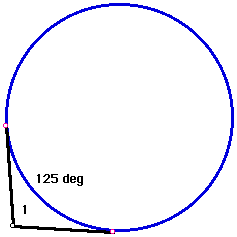
Problems 1-4: Find the measure of ange 1.
1.
2.
3.
4.
5. Stonehenge is an ancient structure in England. The following is a geometric
description of the structure.

a. You are standing at point A. What is the measure of angle BAC
b. Suppose B and C were moved so that line AB and line AC are tangents.
Wound the measure of
angle BAC be larger or smaller?
I will have the students work together in groups and present their solutions
to the class.































