![]()
More particularly this results in b = sqrt (ac) and b is called the mean proportional. The construction of the mean proportional relies on an understanding of similar triangles.
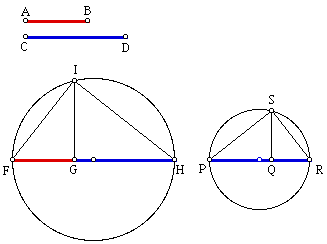
In the left hand construction FG is equal to AB and GH is equal to CD, FH is the diameter of the circle and GI is perpendicular to FH. The following triangles are similar: FGI, FIH and IGH, from where it follows that:
![]()
and IG is the mean proportion between AB and CD.
In the right hand construction, PQ is equal to AB and PR is equal to CD, PR is the diameter of the circle and SQ is perpendicular to PR. The following triangles are similar: PQS, PSR and SQR, from where it follows that:
![]()
and PS is the mean proportional between AB and CD.