Outcomes of Connecting Consecutive Midpoints
by: Marcia Bailey, Linda Crawford, and Cynthia Dozier
__________________________________________________________
Consider the outcome of taking any quadrilateral and connecting consecutive midpoints of the sides. What kind of figure is formed? What if you did the same routine with specific types of quadrilaterals? What figure is formed by joining the midpoints of a rectangle, kite, isosceles trapezoid, square or a rhombus? Let's first look at each case and then try to explain some conclusions on how you can predict the outcome of other specific cases ?
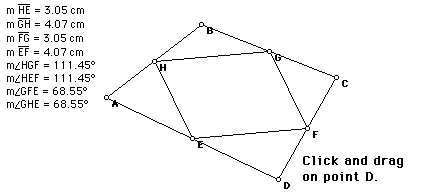
Case 1. Take any quadrilateral. As you can see from the sketch below, the figure formed is a parallelogram. By measuring opposite sides and angles and showing them congruent, we know the figure must be a parallelogram by definition. If you click here, you can change the quadrilateral to other various shapes and sizes to show the conclusion holds. The secondary figure will always continue to be a parallelogram.

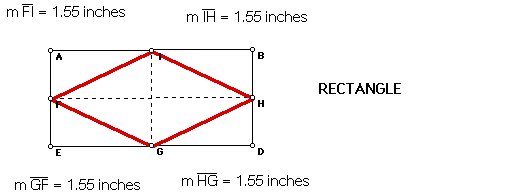
Case 2. If you repeat the process for a rectangle and an isosceles trapezoid, the figures formed appear to each be a rhombus,as shown in each of the figures below. This is proven by measuring all four sides of each secondary figure and showing that each has four congruent sides. By definition, this shows that the secondary figure is a rhombus.

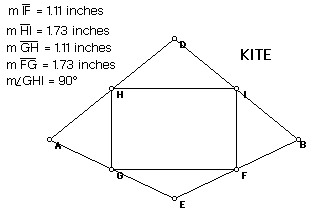
Case 3. In a kite, the construction below illustrates that the secondary figure is a rectangle. This is shown by measuring opposite sides and showing that the oppositie sides are congruent. Also, all four angles are right angles. By clicking here, you can move each vertex of the kite and the secondary figure continues to always be a rectangle no matter the size of the kite or the length of each segment.
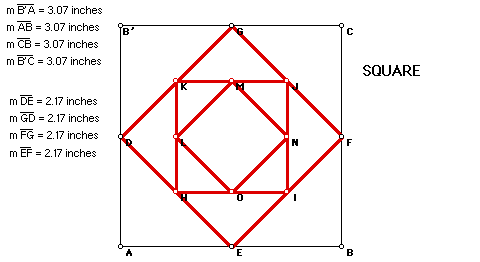
Case 4. The square and the parallelogram each have a fascinating characteristic in their constructed secondary figures. When connecting the consecutive midpoints on each of these separate quadrilaterals, the figure formed is a square for the square and a parallelogram for the parallelogram. As you can see below, even after several iterations of the process, each figure formed is an image of itself

Case 5. Interestingly, the rhombus will produce a rectangle when connecting consecutive midpoints. Previously we showed the reverse of this. When we connected the midpoints of the rectangle, we formed a rhombus. Notice the sequence below as the process is repeated over and over again.
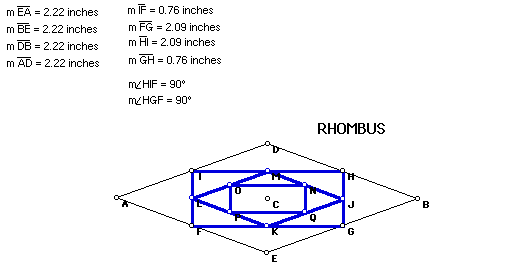
Summary. There were several conclusions that were found to help in predicting the type of figure that would be formed. Some of those conclusions follow. Each of these conclusions relates to one of the cases presented previously and its validity holds for each one. First, when the diagonals of the original figure are perpendicular, the secondary figure will have right angles. Think about the rhombus, rectangle and square. What types of figures were formed and were their diagonals perpendicular? Second, if the segments connecting the midpoints of the sides of the original figure were perpendicular bisectors, then four congruent triangles were formed. Last, if segments connecting midpoints bisect each other, then the opposite sides of the secondary figure are congruent.
Return to Marcia's home page .