By: Fhonda Danley & September Matteson

EMT 469 - WTR 96

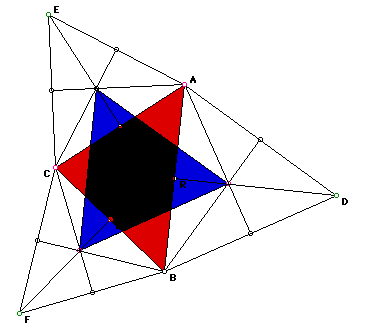
Starting with the red triangle, ABC, we constructed three equilateral triangles on the exterior of ABC. Next, we found the centers of each of these equilateral triangles and joined these centers to form the blue triangle. This triangle can also be known as Napoleon's triangle.

Our question now is whether or not this blue triangle is indeed Napoleon's triangle. One easy way to investigate this would be to measure the sides of the blue triangle and see if they are congruent, however; this would only give us a hypothesis and not an actual proof. So, let's try this and see if we get this result for a hypothesis.

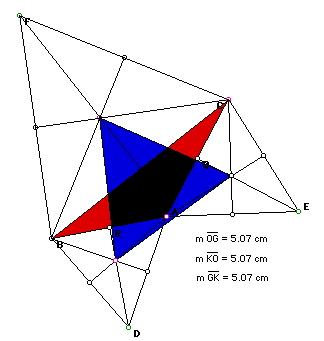
As we can see, from the picture, all of the sides are indeed congruent, giving us 5.07 cm for the length. Now we can give a hypothesis.

Hypothesis: Given any type of triangle ABC, whenever we construct three equilateral triangles on each side of the original, if we join the centers of these triangles our newly formed triangle will be a Napoleon's triangle.

Now, let's investigate this using different types of triangles as our triangle ABC.

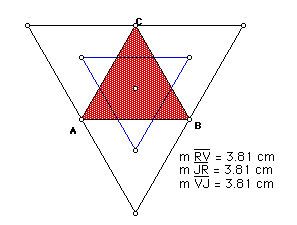
For this picture, above, we can see what happens when our triangle ABC is an equilateral triangle. Our result is the blue triangle where once again all of the sides are congruent. Now let's see if we get the same results when our triangle ABC is isosceles.

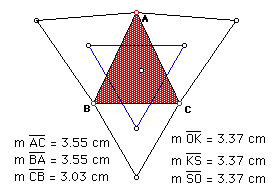
From this, we see what happens when our ABC is isosceles(its measurements are shown on the left side of the picture). We see that the sides of our blue triangle are indeed congruent once again. Let's investigate what happens when the triangle is right triangle.

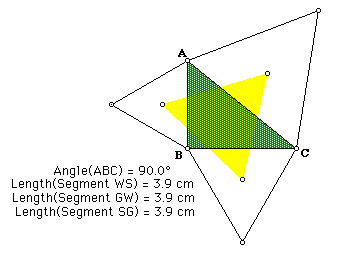
As we can see, once again our newly formed triangle, yellow triangle, has all lengths congruent. Therefore, by definition of Napoleon's triangle, it is indeed.

Now, I think that we can safely say that by way of our hypothesis, we have proven what we set out to prove. That yes indeed whenever you have any given triangle where three equilateral triangles are formed on its exterior, when the centers of these triangles (equilateral) are joined, the resulting triangle will be a Napoleon's triangle (where all of its sides are congruent).