How Parametric Equations of the FormHow Parametric Equations of the Form

x = t + a

y = kt + b

Lead to Linear Equations of the Form

y = mx + c

by

June Jones

EMT 668

Fall 1995

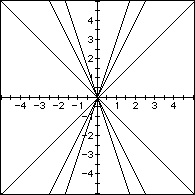
It is often advantageous to take the rather complicated equation of a relation in rectangular form and rewrite it in parametric form. Since most graphing applications, other than Algebra Xpresser, don't allow for direct entry of a relation, the change to parametric makes it possible to graph. For this reason, it is usually only the curves that are considered in most treatments of parametric equations. Consider now the relationship between linear equations in parametric form and rectangular form.

Begin with the pair x = t + a, y = kt + b by setting a = 0, b = 0, k = -3, -2, -1, 0, 1, 2, 3

and - t . Observe a family of lines through (0,0) with k equal to the slope if the line.

Thinking perhaps that k = m in general, try setting a = 2, b = 2. Again, we see a family of lines with slope of k through (2,2). Now, let k continue to be represented by the previous values and allow a b. For example, let a = 2 and b = -3. The result is the family of lines with like orientation and intersecting at (2, -3).

It can now be conjectured that m = k and (a,b) is a point of the line. In general, take the parametric form x = t + a and y = kt + b, and the rectangular form y = mx + c and substitute:

(kt + b) = k(t + a) + c

kt + b = kt + ka + c

b = ka + c

b - ka = c

Thus (a, b) is a point on the line, k is the slope of the line, and b - ka is the y-intercept.

Now use the general conclusion for a specific case:

[1] Write parametric equations of a line through (7,5) with slope 3. Graph the line using

your equations.

Solution: (a,b) = (7,5), a point of the line, k = 3, the slope

The parametric equations obtained are: x = t + 7 and y = 3t + 5

It should also be observed that the parametric equations above are not the only pair that will meet the given requirements. For example, x = t + 1 and y = 3t - 13 yield the same line. Thus the form x = t + a, y = kt + b, does not yield a unique pair. One further transformation will result in a unique pair. Since b - ka = c and c represents the y-intercept in rectangular form, set (a,b) = (0,c) = (0,b-ka). Thus the pair x = t, y = 3t - 16 would be the more general. Now x = t and y = kt + c while k is the slope and c the y-intercept.

[2] Write the equation of the line in rectangular form.

Solution: (a,b) = (7,5), m = 3, c = b - ka = 5 - 3(7) = -16

Thus: y = 3x - 16

The relationship between the forms of the line should now be obvious.