A Paradox

in

Geometrical Probability


by

Kyungsoon Jeon and Larry Shook

Introduction

We were working on a group project for EMT 708 in which we examined curriculum development material. High School Mathematics and Its Applications (HiMAP) is a curriculum development project which has produced modules for high school teachers. Applications of Geometrical Probability by Fred C. Djang is one of the modules produced by HiMAP. One of the problems presented in Applications of Geometrical Probability looked very interesting and could be presented in the mathematics classroom visually using GSP.

The Problem

Given a circle with diameter 2, what is the probability that the length of a chord drawn will exceed ?

In the way the question is posed, there are several different models which could be looked at to examine this problem. Depending on which model is chosen, several different solutions can be found. We will examine three models and the solutions for each of the models presented.

Model 1
 





  
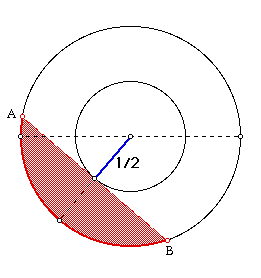
In Model 1, the radius of the inside circle is 1/2 and that of outside circle is 1. 
Thus, we have by the Pythagorean Theorem the length of half of the chord is 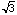/2. 
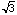So we obtained   as the length ofthe chord. If the chord passes through the inside 
circle, then the length of AB will exceed 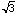.  If the chord does not pass through the 
inside circle, then the length of AB is less than 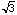. Here,  AB is 
tangent to the inside circle and we  can rotate AB around the inside circle.                                  


With this interpretation, we can define the probability as below.



Probability = Measure of the Feasible Region/Measure of the Sample Region



= Area of the Inner Circle/Area of the Outside Circle



= [[pi]] (1/2)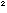/ [[pi]] (1)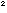 



= 1/4



We obtained 1/4 as a solution. Is this solution sufficient to answer the
problem or might there be other ways to examine the problem? We can think
of another approach in which we can come up with a different solution. Of
course, we need a different model. 



Model 2





 


                                        

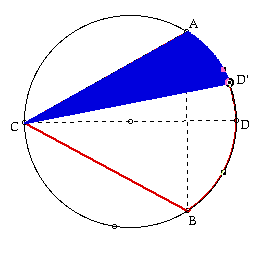
In this model, the endpoint, D', of the chord CD' can be moved along the     
circumference of the circle from the fixed point C.  Since any angle          
inscribed in a semicircle is a right angle, we have that ACD is a right       
triangle.  Thus, if  the angle ACD is  [[pi]]/6, then the angle ADC is          
[[pi]]/3. So, now we can apply the relationship between the ratio of the    
three angles [[pi]]/3, [[pi]]/6, and  [[pi]]/2 and that of the three lengths   
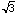, 1, and 2, respectively.  Since the diameter of the circle is 2, we have the length of chord AC is 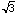.  We have    
the desired probability when the chord CD' moves along the arc ADB.             


Probability = Measure of the Feasible Region/ Measure of the Sample Region



= Length of Arc ADB/ Circumference of the Circle



= (2/3)[[pi]]/2[[pi]] 



=1/3 



We obtained a different solution compared with Model 1. But we can not tell
which one is right or wrong because the models that we examined are different.
Now let us examine one last model.



Model 3







                                           
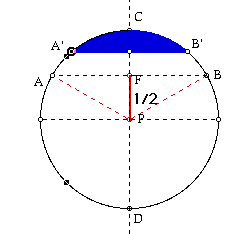
In this model, the chord A'B' is perpendicular to the diameter CD.  As    
the chord A'B' moves from the point C and down to the chord AB. Since the      
length of PB is 1 and length of FP is1/2 the length of the chord A'B' is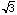.  The
Pythagorean Theorem gives us BF is 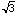/2 and hence AB is 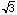.  The desired probability 
is obtained when the chord   A'B' starts moving from AB to the point P.                                 


With this interpretation, we can define the probability as below.



Probability = Measure of the Feasible Region/ Measure of the Sample Space



= Length of FP/Length of CP 



= (1/2)/1 



In examining this problem, choosing the diameter of the circle to be 2 and
the length of the chord to be the makes the problem accessible to students.
Students can use their knowledge of high school geometry in solving the
problem. As the parameters of the problem are changed in each model, the
nature of the solution changes. Students are encouraged to find other approaches
which generate other solutions. Teachers can use this idea to develop extensions
to this problem.