We began the investigation of several classical curves, limacon, cardioid, lemniscate and the spiral of Achimedes. We were investigating these curves in Geometer's Sketch Pad to discover if their construction would help to describe the curves more fully since curves that are illustrated in Polar coordinates for high school students are sometimes difficult to comprehend since they spend most of their time in the Cartesian coordinate system.

We used Mathematics Teacher Workshop to describe the basic curves in their Polar form beginning with the spiral of Achimedes.

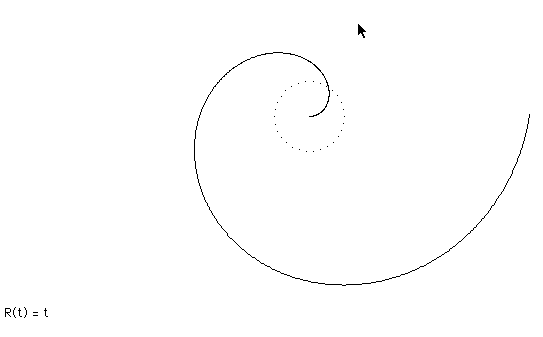
We were able to construct a version of the spiral of Archimedes that animates using Geometer's Sketch Pad it would do the investigator well to go into the animation and display all hidden to get an idea of the construction and how using the construction along with the animation might explain the effects of ( t ) (the angle) and ( r ) (the radius) on the generated curve.

Our next investigation was of the limacon. A curve discovered by Etienne Pascal (1588 - 1640). This curve is defined as the set of all points whose distance from the circle C measured along a line through the origin is constantly equal to 1, the radius of C , when the circle C is centered at (1,0). Using the polar equation r = a + bcos(t) where a = 1 and b = 2 we generate the curve:

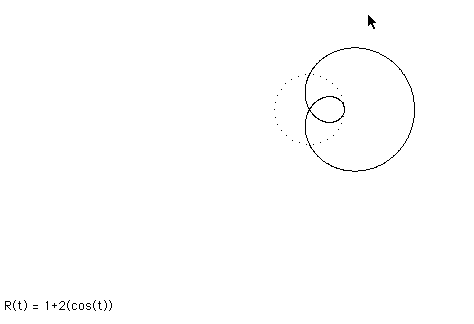
We can see in the animation that by tracing the locus of two points we generate the limacon and a circle of the same size as C that is centered around the origin. The limacon can be cleaned up to illustrate only the attractive "snail" curve.

The next curve we investigated, the cardioid is a special case of the limacon where a = b , and in our case a = b = 1

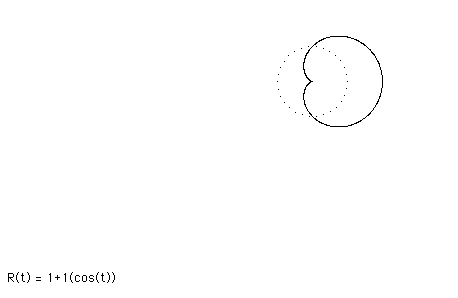
A significant difference that is discovered through the construction of the cardioid is that the circle generated when the locus of the points are traced has a radius that is the diameter of the original circle C. And, again the caridoidcurves construction can be cleaned up to generate only the desired "heart" curve.

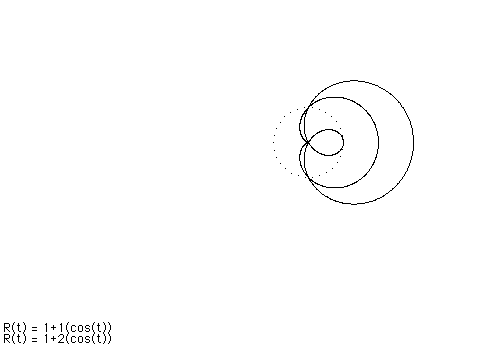
When we overlay the cardioid and the limacon a difference can be observed although it is impossible to differentiate where the cusp of the cardioid and the loop of the limacon of the separate curves are located.

We illustrated both the limacon and the cardioid in cosine only, they can both be illustrated in sine which causes a ninety degree rotation for either curve. However, the last curve we investigated only has a forty-five degree rotation when you change from sine to cosine. Below we illustrated the lemniscate, r^2 = a^2cos (2t) where a = 2.

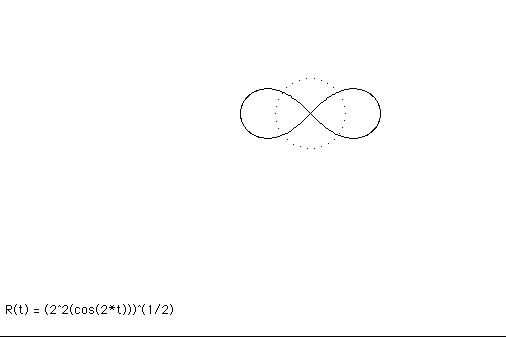
And then we illustrated the same curve in sine, r^2 = a^2sin(2t).

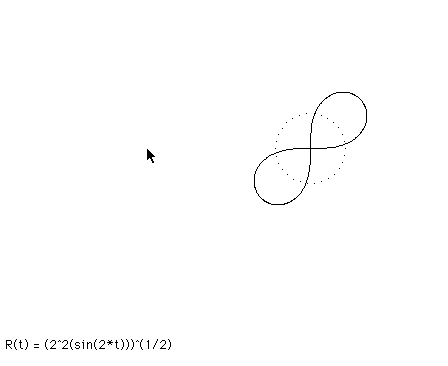
We constructed the animation for thelemniscate in a slightly different manner, using an envelope of circles to describe the curve verses tracing locus of points.

These classical curves were exciting investigations in Geometer's Sketch Pad, and provided assistance in describing the curve based on their construction. Other classical curves that would be worthy of investigation might be the "witch" of Agnesi, and the cycloid.