PROBLEM: Arithmetic Mean -- Geometric Mean Inequality

Find 5 different demonstrations (proofs) of the Arithmetic Mean -- Geometric Mean inequality for two non-negative numbers

One example.

Another suggestion.

Some Algebra.

Another example.

And still another example . . .

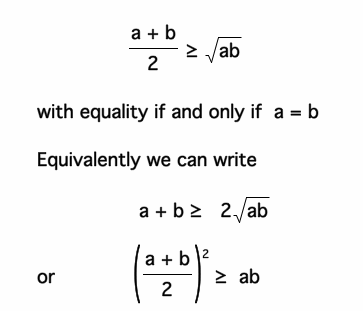
More algebra . . .

Using some geometry . . .

One More with Algebra

Using a family of curves xy = c and a line x + y = 2m

Can you generalize this theorem?

In the case of three positive quantities:

In general,

For a discussion of one proof of these generalizations, see Courant, R,. & Robbins, H. (1941) What is Mathematics? New York: Oxford University Press, pp. 361-365.