Ask student to use GSP to answer the following questions:
In the typical high school algebra I and II courses students generally
study quadratic functions of the form ![]() . They find the axis of symmetry,
vertex and maximum or minimum value of the function. They rarely discuss
the construction of the parabola as a conic section and generally do not
understand its geometric construction. This write up will discuss the ways
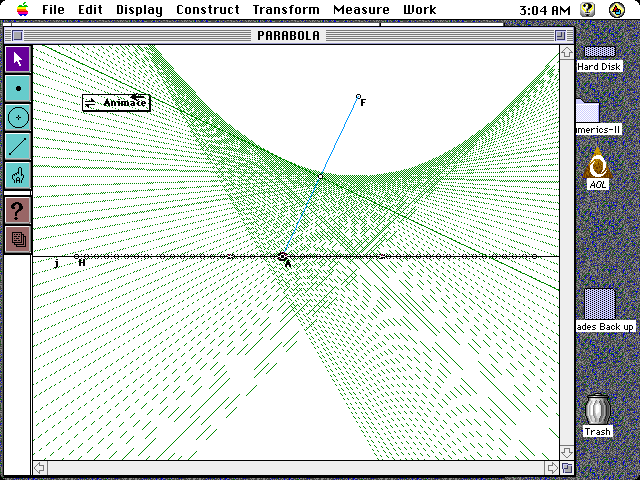
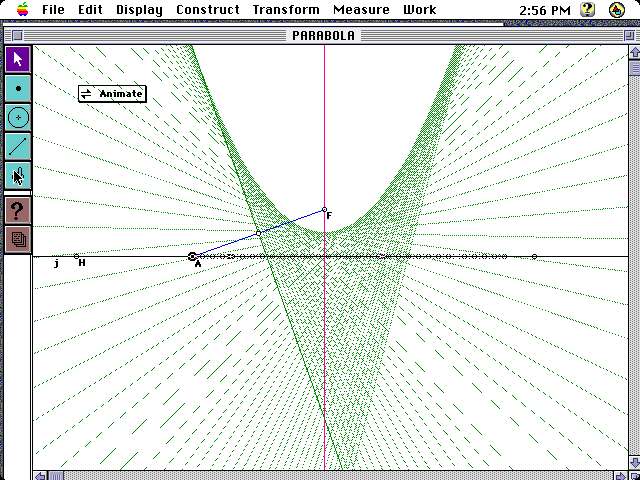
a student might construct the parabola given a line and a point. A parabola
is a set of points equidistant from a line, called the directrix, and a
fixed point called the focus. Assume the focus is not on the line. One might
begin by having students do a paper folding activity. Using any type of
paper draw a line j on your paper. This will be the directrix. Chose an
arbitrary point F on the paper to represent the focus. Instruct the students
to fold the paper in such a way that F falls on line j. After making several
folds they should begin to see the form of a parabola taking place. This
should include a discussion of the perpendicular bisector being formed.
Follow up with a Geometer's Sketch Pad lab in which they construct the parabola
with similar reasoning. See a GSP diagram by clicking HERE
. They find the axis of symmetry,
vertex and maximum or minimum value of the function. They rarely discuss
the construction of the parabola as a conic section and generally do not
understand its geometric construction. This write up will discuss the ways
a student might construct the parabola given a line and a point. A parabola
is a set of points equidistant from a line, called the directrix, and a
fixed point called the focus. Assume the focus is not on the line. One might
begin by having students do a paper folding activity. Using any type of
paper draw a line j on your paper. This will be the directrix. Chose an
arbitrary point F on the paper to represent the focus. Instruct the students
to fold the paper in such a way that F falls on line j. After making several
folds they should begin to see the form of a parabola taking place. This
should include a discussion of the perpendicular bisector being formed.
Follow up with a Geometer's Sketch Pad lab in which they construct the parabola
with similar reasoning. See a GSP diagram by clicking HERE
Ask student to use GSP to answer the following questions:

To examine the relationship between the parabola and its equation we could
use the coordinate plane and the distance formula to develop an equation.
Example: Determine an equation of the parabola that is equidistant from
y = 0 and the point (0,2).
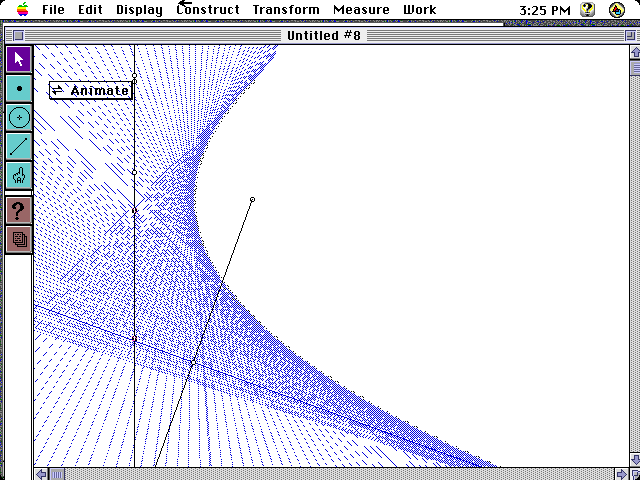
Solution: Let P be a general point that is equidistant from y = 0
and ( 0,2) . See diagram below.
Distance 1 (d1) = y - 0
Distance 2 ( d2) = ![]()
Since d1 = d2, then
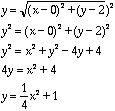
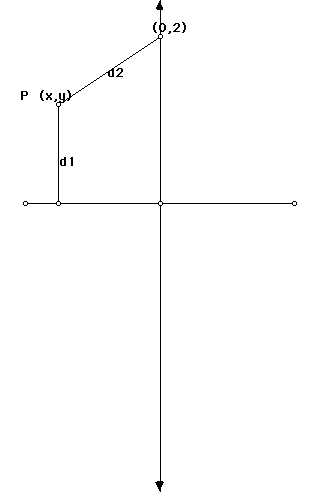
After the equation is developed then a natural discussion could take place
about the effects of the coefficient of the squared term and the constant
term in the equation. The discussion might extend on into other conics and
their constructions.
RETURN TO SANDY'S HOME PAGE