

Problem Set 2.1
1. a. State and prove an 'if and only if' condition relating two congruent chords in and circle and their distances from the center of the circle. Make sure your statement and proof applies to the following cases:
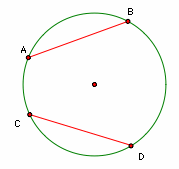
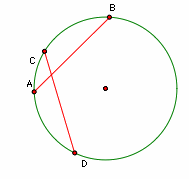
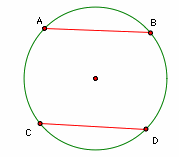
Theorem: Two chords of the same circle are congruent iff they are the same distance from the center.
Proof: If AB = CD, construct isosceles triangles AOB and COD. The altitudes AB and CD form pairs of right triangles. They are all congruent by the HL congruence condition. Therefore the altitudes have the same length and the chords are the same distance from the center.
If the altitudes are of equal length, the triangles cans once again be shown to be congruent by the HL Congruence condition and that can lead to AB = CD by combining the congruent sides of the triangles.
b. Given two chords in a circle, prove that the longer chord is closer to the center of the circle. Watch out for all cases.
Given AB < CD, prove OE > OF. See GSP File
ALTERNATIVE PROOF for 2.1.1b
Consider this figure and construct CD congruent to AB (OE is congruent to OG also).
(proof to be developed by a volunteer)
c. The converse: Given OE > OF, prove AB < CD.
2. State and prove the converse of Proposition 2.3. If a diameter bisects a chord and bisects each of the arcs determined by the chord, then the diameter is perpendicular to the chord.
See GSP file. Just a drawing to suggest a proof ...
See Write-up by Brenda King (GSP file)
3.a. Prove that the tangent to a circle intersects the circle in only one point. (See Now Solve This 2.1) Open GSP File.
3.b. Prove that the line that intersects the circle in exactly one point is tangent to the circle.
Look at the definition of a tangent to a circle. It says "A tangent to a circle O at point P on the circle is the line through P, perpendicular to OP." This problem then is:
Given a line that intersects the circle O at exactly one point P, prove that OP is perpendicular to the line.
4, 5, and 6. Alternative proofs of the Inscribed Angle Theorem.
7. Open GSP file -- just the drawings
In each of the following cases, if possible find unique values for x or for x, y, and z. If not possible prove that there are infinitely many solutions. (O is the center of each circle.)
8. Open GSP File.
Given a triangle ABC inscribed in a circle with Orthocententer at H. Extend the altitude AD to the intersection with the circumscribe at K. Prove that HD = DK.
9. a. Show the angle formed by the intersection of two secants on the interior of the circle in terms of the intercepted arcs.
Hint: Construct line AD. Consider the triangle AED. Angle BEA is an external angle of the triangle and the remote interior angles can be expressed in terms of the intercepted arcs AB and CD.
Solution by Anne Marie Marshall (GSP File)
9.b. Show the angle formed by the intersection of two secants on the exterior of the circle in terms of the intercepted arcs.
Hint: We need at least one auxiliary segment. Try two: AC and BD. Now, angle DBC is an external angle of triangle DBE.
Solution by Anne Marie Marshall (GSP File)
Discuss the results in terms of the Inscribed Angle theorem being a special case of either.
10. Prove that two parallel chords in a circle create congruent arcs. This is the same thing as showing segments AB and CD
Hint: The segment AC would be a transversal of the two parallel chords. Alternate interior angles CAD and ACB would be of equal measure and each subtends one of the arcs of interest.
Solution by Anne Marie Marshall (GSP file)
11. Use result of problem 10 to solve problem 9a.
12. a. What kind of parallelograms are cyclic? Justify.
b. What kinds of trapezoids are cyclic? Justify.
13.
Hint: The measure of angle CAB is the measure of the angle formed by line m and CB. But CB is a transversal of two parallel lines. So the angle line n makes with CB is also congruent to angle CAB. Thus angle DEB + angle CAB = 180 degrees and ABED is cyclic.
14. Triangle ABC is inscribed in circle O. The altitude BH intersects the diameters through A and C respectively.
a. Prove triangle DOE is isosceles. Open GSP file?
b. Will it hold if ABC is an obtuse triangle? NOTE: Extend the sides of triangle ABC outside the circle.
15.Segment AB is a diameter of a semicircle with center O. Let S be on the ray AB, and let the line SC be tangent to the semicircle. If ST bisects angle ASC and intersects arc AC at D, find the measure of angle STC. Justify. Open GSP File?
16. Given a circle with center O and a point A external to the circle. Let B and C be the points of tangency of the tangents drawn from A to the circle, and let CP be a diameter of the circle. Construct the line PB and locate its intersection E with line CA.
Prove that the measure of Angle BAC is twice the measure of angle BEC. A figure is in the GSP file, but you should try to draw your own figure first. Hint for the proof? Try it first.
17. ABCD is inscribed in a circle O. AB is a diameter, and E is the intersection of lines along opposite sides AD and BC. Construct OD. For the second part of the problem consider the case where AB is not diameter.
a. When AB is a diameter, prove angle ODC is congruent to angle AEB.
b. Prove that line OD is tangent to the circle that circumscribes triangle DEC.
c. Which of the above holds when AB is not a diameter?
Do a GSP sketch where segment AB is not necessarily a diameter and drag one end of the segment along the arc of the circle. Why do each of a) and b) hold or not hold?